We give a new factorisation of the classical Dynkin operator, an element of the integral group ring of the symmetric group that facilitates projections of tensor powers onto Lie powers. As an application we show that the iterated Lie power 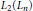${{L}_{2}}({{L}_{n}})$ is a module direct summand of the Lie power 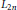${{L}_{2n}}$ whenever the characteristic of the ground field does not divide $n$. An explicit projection of the latter onto the former is exhibited in this case.