Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Henriot, Kevin
2014.
On Arithmetic Progressions in A+B+C.
International Mathematics Research Notices,
Vol. 2014,
Issue. 18,
p.
5134.
Shkredov, Ilya Dmitrievich
2015.
Структурные теоремы в аддитивной комбинаторике.
Успехи математических наук,
Vol. 70,
Issue. 1(421),
p.
123.
Croot, Ernie
Lyall, Neil
and
Rice, Alex
2015.
Polynomials and Primes in Generalized Arithmetic Progressions.
International Mathematics Research Notices,
Vol. 2015,
Issue. 15,
p.
6021.
Hatami, Hamed
Hosseini, Kaave
and
Lovett, Shachar
2016.
Structure of Protocols for XOR Functions.
p.
282.
Bloom, T. F.
2016.
A quantitative improvement for Roth's theorem on arithmetic progressions: Table 1..
Journal of the London Mathematical Society,
Vol. 93,
Issue. 3,
p.
643.
Henriot, Kevin
2016.
ARITHMETIC PROGRESSIONS IN SETS OF SMALL DOUBLING.
Mathematika,
Vol. 62,
Issue. 2,
p.
587.
SCHOEN, TOMASZ
and
SISASK, OLOF
2016.
ROTH’S THEOREM FOR FOUR VARIABLES AND ADDITIVE STRUCTURES IN SUMS OF SPARSE SETS.
Forum of Mathematics, Sigma,
Vol. 4,
Issue. ,
Hatami, Hamed
Hosseini, Kaave
and
Lovett, Shachar
2018.
Structure of Protocols for XOR Functions.
SIAM Journal on Computing,
Vol. 47,
Issue. 1,
p.
208.
Sanders, Tom
2020.
Bounds in Cohen’s Idempotent Theorem.
Journal of Fourier Analysis and Applications,
Vol. 26,
Issue. 2,
Schoen, Tomasz
2021.
О суммах множеств квадратов.
Труды Математического института имени В. А. Стеклова,
Vol. 314,
Issue. ,
p.
311.
Schoen, Tomasz
2021.
On Sumsets of Subsets of Squares.
Proceedings of the Steklov Institute of Mathematics,
Vol. 314,
Issue. 1,
p.
300.
Hanson, Brandon
2021.
Long regularly-spaced and convex sequences in dense sets of integers.
Journal of Combinatorial Theory, Series A,
Vol. 180,
Issue. ,
p.
105393.
Sanders, Tom
2021.
Coset decision trees and the Fourier algebra.
Journal d'Analyse Mathématique,
Vol. 144,
Issue. 1,
p.
227.
Semchankau, Aliaksei
Shabanov, Dmitry
and
Shkredov, Ilya
2022.
Number of A+B≠C solutions in abelian groups and application to counting independent sets in hypergraphs.
European Journal of Combinatorics,
Vol. 100,
Issue. ,
p.
103453.
Ramaré, Olivier
2024.
An Explicit Croot-Łaba-Sisask Lemma Free of Probabilistic Language.
Bulletin of the Brazilian Mathematical Society, New Series,
Vol. 55,
Issue. 2,
HUNTER, ZACH
and
POHOATA, COSMIN
2025.
On off-diagonal Ramsey numbers for vector spaces over
$\mathbb{F}_{2}$
.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 179,
Issue. 2,
p.
503.
Semchankau, Aliaksei
2025.
A new bound for A(A + A) for large sets.
Journal of Number Theory,
Vol. 268,
Issue. ,
p.
142.
 \begin{equation}\exp ( c (\alpha \beta \log N)^{1/2} - \log\log N).\end{equation}
\begin{equation}\exp ( c (\alpha \beta \log N)^{1/2} - \log\log N).\end{equation}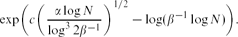 \begin{equation}\exp\biggl( c \biggl(\frac{\alpha \log N}{\log^3 2\beta^{-1}} \biggr)^{1/2} - \log( \beta^{-1} \log N) \biggr).\end{equation}
\begin{equation}\exp\biggl( c \biggl(\frac{\alpha \log N}{\log^3 2\beta^{-1}} \biggr)^{1/2} - \log( \beta^{-1} \log N) \biggr).\end{equation}
