Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Jiang, Tao
and
Ma, Jie
2018.
Cycles of given lengths in hypergraphs.
Journal of Combinatorial Theory, Series B,
Vol. 133,
Issue. ,
p.
54.
Ergemlidze, Beka
Győri, Ervin
and
Methuku, Abhishek
2019.
Asymptotics for Turán numbers of cycles in 3-uniform linear hypergraphs.
Journal of Combinatorial Theory, Series A,
Vol. 163,
Issue. ,
p.
163.
Mubayi, Dhruv
and
Wang, Lujia
2019.
The Number of Triple Systems Without Even Cycles.
Combinatorica,
Vol. 39,
Issue. 3,
p.
679.
Méroueh, Arès
2019.
The Ramsey number of loose cycles versus cliques.
Journal of Graph Theory,
Vol. 90,
Issue. 2,
p.
172.
Jiang, Tao
and
Yepremyan, Liana
2020.
Supersaturation of even linear cycles in linear hypergraphs.
Combinatorics, Probability and Computing,
Vol. 29,
Issue. 5,
p.
698.
Balogh, József
and
Li, Lina
2020.
On the number of linear hypergraphs of large girth.
Journal of Graph Theory,
Vol. 93,
Issue. 1,
p.
113.
Gao, Guorong
and
Chang, An
2021.
A linear hypergraph extension of the bipartite Turán problem.
European Journal of Combinatorics,
Vol. 93,
Issue. ,
p.
103269.
Gyárfás, András
Ruszinkó, Miklós
and
Sárközy, Gábor N.
2022.
Linear Turán numbers of acyclic triple systems.
European Journal of Combinatorics,
Vol. 99,
Issue. ,
p.
103435.
Khormali, Omid
and
Palmer, Cory
2022.
Turán numbers for hypergraph star forests.
European Journal of Combinatorics,
Vol. 102,
Issue. ,
p.
103506.
Gao, Guorong
Chang, An
and
Hou, Yuan
2022.
Spectral Radius on Linear $r$-Graphs without Expanded $K_{r+1}$.
SIAM Journal on Discrete Mathematics,
Vol. 36,
Issue. 2,
p.
1000.
Imolay, András
Karl, János
Nagy, Zoltán Lóránt
and
Váli, Benedek
2022.
Multicolor Turán numbers.
Discrete Mathematics,
Vol. 345,
Issue. 9,
p.
112976.
Gyárfás, András
and
Sárközy, Gábor N.
2022.
The linear Turán number of small triple systems or why is the wicket interesting?.
Discrete Mathematics,
Vol. 345,
Issue. 11,
p.
113025.
Zhang, Fangfang
and
Chen, Yaojun
2022.
The Ramsey Number of 3-Uniform Loose Path Versus Star.
Graphs and Combinatorics,
Vol. 38,
Issue. 1,
Nie, Jiaxi
and
Verstraëte, Jacques
2022.
Ramsey Numbers for Nontrivial Berge Cycles.
SIAM Journal on Discrete Mathematics,
Vol. 36,
Issue. 1,
p.
103.
Qian, Bingchen
Wang, Xin
Xie, Chengfei
and
Ge, Gennian
2023.
Covering Grassmannian Codes: Bounds and Constructions.
IEEE Transactions on Information Theory,
Vol. 69,
Issue. 6,
p.
3748.
Gao, Guorong
Chang, An
and
Sun, Qi
2023.
Asymptotic Turán number for linear 5-cycle in 3-uniform linear hypergraphs.
Discrete Mathematics,
Vol. 346,
Issue. 1,
p.
113128.
Jiang, Tao
Ma, Jie
and
Yepremyan, Liana
2023.
Linear cycles of consecutive lengths.
Journal of Combinatorial Theory, Series B,
Vol. 163,
Issue. ,
p.
1.
Wang, Changxin
and
Zhang, Junxue
2024.
Linear saturation numbers of Berge-C3 and Berge-C4.
Applied Mathematics and Computation,
Vol. 474,
Issue. ,
p.
128685.
Zhang, Lin-peng
and
Wang, Li-gong
2025.
The Linear Turán Numbers of Acyclic Linear 4-graphs.
Acta Mathematicae Applicatae Sinica, English Series,
Zhou, Junpeng
and
Yuan, Xiying
2025.
Turán problems for star-path forests in hypergraphs.
Discrete Mathematics,
Vol. 348,
Issue. 11,
p.
114592.
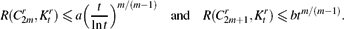 \begin{equation}R(C^r_{2m}, K^r_t)\leq a \Bigl(\frac{t}{\ln t}\Bigr)^{{m}/{(m-1)}}\quad\text{and}\quadR(C^r_{2m+1}, K^r_t)\leq b t^{{m}/{(m-1)}}.\end{equation}
\begin{equation}R(C^r_{2m}, K^r_t)\leq a \Bigl(\frac{t}{\ln t}\Bigr)^{{m}/{(m-1)}}\quad\text{and}\quadR(C^r_{2m+1}, K^r_t)\leq b t^{{m}/{(m-1)}}.\end{equation}
