Article contents
Unavoidable patterns in locally balanced colourings
Published online by Cambridge University Press: 01 June 2023
Abstract
Which patterns must a two-colouring of 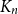 $K_n$ contain if each vertex has at least
$K_n$ contain if each vertex has at least 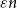 $\varepsilon n$ red and
$\varepsilon n$ red and 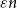 $\varepsilon n$ blue neighbours? We show that when
$\varepsilon n$ blue neighbours? We show that when 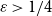 $\varepsilon \gt 1/4$,
$\varepsilon \gt 1/4$, 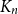 $K_n$ must contain a complete subgraph on
$K_n$ must contain a complete subgraph on 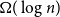 $\Omega (\log n)$ vertices where one of the colours forms a balanced complete bipartite graph.
$\Omega (\log n)$ vertices where one of the colours forms a balanced complete bipartite graph.
When 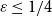 $\varepsilon \leq 1/4$, this statement is no longer true, as evidenced by the following colouring
$\varepsilon \leq 1/4$, this statement is no longer true, as evidenced by the following colouring 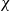 $\chi$ of
$\chi$ of 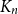 $K_n$. Divide the vertex set into
$K_n$. Divide the vertex set into 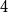 $4$ parts nearly equal in size as
$4$ parts nearly equal in size as 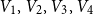 $V_1,V_2,V_3, V_4$, and let the blue colour class consist of the edges between
$V_1,V_2,V_3, V_4$, and let the blue colour class consist of the edges between 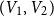 $(V_1,V_2)$,
$(V_1,V_2)$,  $(V_2,V_3)$,
$(V_2,V_3)$, 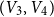 $(V_3,V_4)$, and the edges contained inside
$(V_3,V_4)$, and the edges contained inside  $V_2$ and inside
$V_2$ and inside  $V_3$. Surprisingly, we find that this obstruction is unique in the following sense. Any two-colouring of
$V_3$. Surprisingly, we find that this obstruction is unique in the following sense. Any two-colouring of  $K_n$ in which each vertex has at least
$K_n$ in which each vertex has at least 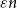 $\varepsilon n$ red and
$\varepsilon n$ red and  $\varepsilon n$ blue neighbours (with
$\varepsilon n$ blue neighbours (with  $\varepsilon \gt 0$) contains a vertex set
$\varepsilon \gt 0$) contains a vertex set 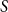 $S$ of order
$S$ of order 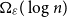 $\Omega _{\varepsilon }(\log n)$ on which one colour class forms a balanced complete bipartite graph, or which has the same colouring as
$\Omega _{\varepsilon }(\log n)$ on which one colour class forms a balanced complete bipartite graph, or which has the same colouring as  $\chi$.
$\chi$.
Keywords
MSC classification
Information
- Type
- Paper
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
Footnotes
Research supported by the European Union’s Horizon 2020 research and innovation programme [MSCA GA No 101038085].
References
- 1
- Cited by


