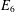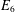Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Fakhruddin, Najmuddin
Khare, Chandrashekhar
and
Patrikis, Stefan
2021.
Relative deformation theory, relative Selmer groups, and lifting irreducible Galois representations.
Duke Mathematical Journal,
Vol. 170,
Issue. 16,