Article contents
GENERIC UNLABELED GLOBAL RIGIDITY
Published online by Cambridge University Press: 30 July 2019
Abstract
Let 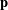 $\mathbf{p}$ be a configuration of
$\mathbf{p}$ be a configuration of  $n$ points in
$n$ points in  $\mathbb{R}^{d}$ for some
$\mathbb{R}^{d}$ for some  $n$ and some
$n$ and some  $d\geqslant 2$. Each pair of points has a Euclidean distance in the configuration. Given some graph
$d\geqslant 2$. Each pair of points has a Euclidean distance in the configuration. Given some graph 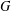 $G$ on
$G$ on  $n$ vertices, we measure the point-pair distances corresponding to the edges of
$n$ vertices, we measure the point-pair distances corresponding to the edges of  $G$. In this paper, we study the question of when a generic
$G$. In this paper, we study the question of when a generic  $\mathbf{p}$ in
$\mathbf{p}$ in  $d$ dimensions will be uniquely determined (up to an unknowable Euclidean transformation) from a given set of point-pair distances together with knowledge of
$d$ dimensions will be uniquely determined (up to an unknowable Euclidean transformation) from a given set of point-pair distances together with knowledge of 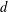 $d$ and
$d$ and  $n$. In this setting the distances are given simply as a set of real numbers; they are not labeled with the combinatorial data that describes which point pair gave rise to which distance, nor is data about
$n$. In this setting the distances are given simply as a set of real numbers; they are not labeled with the combinatorial data that describes which point pair gave rise to which distance, nor is data about 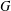 $G$ given. We show, perhaps surprisingly, that in terms of generic uniqueness, labels have no effect. A generic configuration is determined by an unlabeled set of point-pair distances (together with
$G$ given. We show, perhaps surprisingly, that in terms of generic uniqueness, labels have no effect. A generic configuration is determined by an unlabeled set of point-pair distances (together with 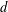 $d$ and
$d$ and  $n$) if and only if it is determined by the labeled distances.
$n$) if and only if it is determined by the labeled distances.
MSC classification
Information
- Type
- Research Article
- Information
- Creative Commons
- This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
- Copyright
- © The Author(s) 2019
References
- 5
- Cited by

