Hostname: page-component-cb9f654ff-mnl9s Total loading time: 0 Render date: 2025-07-31T20:31:35.288Z Has data issue: false hasContentIssue false
  • English
  • Français

Motivic decomposition of anisotropic varieties of type F4 into generalized Rost motives

Published online by Cambridge University Press:  28 May 2008

S. Nikolenko ,
N. Semenov  and
K. Zainoulline
S. Nikolenko
Affiliation:
Department of the Steklov Mathematical Institute, 27, Fontanka, St. Petersburg 191023, Russia
N. Semenov
Affiliation:
Mathematisches Institut, Universitaet Muenchen, Theresienstr. 39, 80333 Muenchen, Germany, semenov@mathematik.uni-muenchen.de.
K. Zainoulline
Affiliation:
Mathematisches Institut, Universitaet Muenchen, Theresienstr. 39, 80333 Muenchen, Germany, kirill@mathematik.uni-muenchen.de.
Get access

Abstract

We prove that the Chow motive of an anisotropic projective homogeneous variety of type F4 is isomorphic to the direct sum of twisted copies of a generalized Rost motive. In particular, we provide an explicit construction of a generalized Rost motive for a generically splitting variety for a symbol in 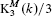. We also establish a motivic isomorphism between two anisotropic non-isomorphic projective homogeneous varieties of type F4. All our results hold for Chow motives with integral coefficients.

Keywords

Chow motivesalgebraic groups of type F4

Information

Type
Research Article
Information
Journal of K-Theory , Volume 3 , Issue 1 , February 2009 , pp. 85 - 102
Copyright
Copyright © ISOPP 2009

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Ar82.Artin, M.. Brauer-Severi varieties. In Dold, A., Eckmann, B. (eds). Lecture Notes in Mathematics 917, Springer-Verlag, Berlin-Heidelberg-New York, 1982, 194–210Google Scholar
Bo03.Bonnet, J.-P.. Un isomorphisme motivique entre deux variétés homogènes projectives sous l'action d'un groupe de type G2. Doc. Math. 8 (2003), 247–277CrossRefGoogle Scholar
Bou.Bourbaki, N.. Lie groups and Lie algebras. Ch. 4–6. Springer-Verlag, Berlin, 2002CrossRefGoogle Scholar
CGM.Chernousov, V., Gille, S., Merkurjev, A.. Motivic decomposition of isotropic projective homogeneous varieties. Duke Math. J. 126 (2005), no. 1, 137–159CrossRefGoogle Scholar
De74.Demazure, M.. Désingularisation des variétés de Schubert généralisées. Ann. Sci. École Norm. Sup. (4) 7 (1974), 53–88Google Scholar
De77.Demazure, M.. Automorphismes et deformations des varieties de Borel. Invent. Math. 39 (1977), no. 2, 179–186Google Scholar
Ful.Fulton, W.. Intersection Theory. Second edition, Springer-Verlag, Berlin-Heidelberg, 1998CrossRefGoogle Scholar
Hi82a.Hiller, H.. Geometry of Coxeter Groups. Research notes in mathematics 54. Pitman Advanced Publishing Program, 1982Google Scholar
Hi82b.Hiller, H.. Combinatorics and intersection of Schubert varieties. Comment. Math. Helv. 57 (1982), no. 1, 47–59Google Scholar
IM05.Iliev, A., Manivel, L.. On the Chow ring of the Cayley plane. Compositio Math. 141 (2005), 146–160Google Scholar
Inv.Knus, M.-A., Merkurjev, A., Rost, M., Tignol, J.-P.. The book of involutions. AMS Colloquium Publications, vol. 44, 1998Google Scholar
IK00.Izhboldin, O., Karpenko, N.. Some new examples in the theory of quadratic forms. Math. Zeitschrift 234 (2000), 647–695Google Scholar
Ka98.Karpenko, N.. Characterization of minimal Pfister neighbors via Rost projectors. J. Pure Appl. Algebra 160 (2001), 195–227Google Scholar
Ka01.Karpenko, N.. Cohomology of relative cellular spaces and isotropic flag varieties. St. Petersburg Math. J. 12 (2001), no. 1, 1–50Google Scholar
Ka04.Karpenko, N.. Holes in In. Ann. Sci. École Norm. Sup. (4) 37 (2004), no. 6, 973–1002Google Scholar
KM02.Karpenko, N., Merkurjev, A.. Rost Projectors and Steenrod Operations. Documenta Math. 7 (2002), 481–493Google Scholar
Ko91.Köck, B.. Chow Motif and Higher Chow Theory of G/P. Manuscripta Math. 70 (1991), 363–372Google Scholar
Ma68.Manin, Y.. Correspondences, Motives and Monoidal Transformations. Math. USSR Sbornik 6 (1968), 439–470CrossRefGoogle Scholar
MT95.Merkurjev, A.S., Tignol, J.-P.. The multipliers of similitudes and the Brauer group of homogeneous varieties. J. Reine Angew. Math. 461 (1995), 13–47Google Scholar
PR94.Petersson, H.P., Racine, M.L.. Albert Algebras. In Kaup, W., McCrimmon, K., Petersson, H.P. (eds). Jordan Algebras. Proc. of the Conference in Oberwolfach, Walter de Gruyter, Berlin-New York, 1994, 197–207Google Scholar
Ro98.Rost, M.. The motive of a Pfister form. Preprint, 1998. http://www.mathematik.uni-bielefeld.de/~rost/Google Scholar
St04.Stembridge, J.. The coxeter and weyl maple packages. 2004. http://www.math.lsa.umich.edu/~jrs/maple.htmlGoogle Scholar
Su05.Suslin, A., Joukhovitsky, S.. Norm Varieties. J. Pure Appl. Algebra 206 (2006), 245–276Google Scholar
Ti66.Tits, J.. Classification of algebraic semisimple groups. In Algebraic Groups and Discontinuous Subgroups (Proc. Symp. Pure Math.), Amer. Math. Soc., Providence, R.I., 1966Google Scholar
Vo03.Voevodsky, V.. On motivic cohomology with ℤ/l-coefficients, Preprint, 2003. http://www.math.uiuc.edu/K-theoryCrossRefGoogle Scholar