Hostname: page-component-848d4c4894-5nwft Total loading time: 0 Render date: 2024-04-30T16:08:36.692Z Has data issue: false hasContentIssue false
  • English
  • Français

Pseudo-jump operators. II: Transfinite iterations, hierarchies and minimal covers

Published online by Cambridge University Press:  12 March 2014

Carl G. Jockusch Jr.  and
Richard A. Shore
Carl G. Jockusch Jr.
Affiliation:
Cornell University, Ithaca, New York 14853
Richard A. Shore
Affiliation:
Cornell University, Ithaca, New York 14853
Get access Rights & Permissions [Opens in a new window]

Extract

In this paper we introduce a new hierarchy of sets and operators which we call the REA hierarchy for “recursively enumerable in and above”. The hierarchy is generated by composing (possibly) transfinite sequences of the pseudo-jump operators considered in Jockusch and Shore [1983]. We there studied pseudo-jump operators defined by analogy with the Turing jump as ones taking a set A to A ⊕ 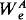for some index e. We would now call these 1-REA operators and will extend them to α-REA operators for recursive ordinals α in analogy with the iterated Turing jump operators (A → A(α) for α < 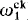and Kleene's hyperarithmetic hierarchy. The REA sets will then, of course, be the results of applying these operators to the empty set. They will extend and generalize Kleene's H sets but will still be contained in the class of 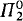set singletons thus providing us with a new richer subclass of the 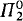set singletons which, as we shall see, is related to the work of Harrington [1975] and [1976] on the problems of Friedman [1975] about the arithmetic degrees of such singletons. Their degrees also give a natural class extending the class H of Jockusch and McLaughlin [1969] by closing it off under transfinite iterations as well as the inclusion of [d, d′] for each degree d in the class. The reason for the class being closed under this last operation is that the REA operators include all 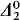operators and so give a new hierarchy for them as well as the 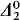sets. This hierarchy also turns out to be related to the difference hierarchy of Ershov [1968], [1968a] and [1970]: every α-r.e. set is α-REA but each level of the REA hierarchy after the first extends all the way through the difference hierarchy although never entirely encompassing even the next level of the difference hierarchy.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 49 , Issue 4 , December 1984 , pp. 1205 - 1236
Copyright
Copyright © Association for Symbolic Logic 1984

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Cooper, S. B. [n.d.], Initial segments of degrees containing non-recursive recursively enumerable degrees, preprint.Google Scholar
Enderton, H. and Putnam, H. [1970], A note on the hyper-arithmetical hierarchy, this Journal, vol. 35, pp. 429–430.Google Scholar
Epstein, R., Haass, R. and Kramer, R. [1981], Hierarchies of sets and degrees below 0′, Logic Year 1979–80 (Lerman, M., Schmerl, J. and Soare, R., editors), Lecture Notes in Mathematics, vol. 859, Springer-Verlag, Berlin, pp. 32–48.CrossRefGoogle Scholar
Ershov, Yu. L. [1968], A hierarchy of sets. I, Algebra i Logika, vol. 7, no. 1, pp. 47–74; English translation, Algebra and Logic, vol. 7, pp. 25–43.Google Scholar
Ershov, Yu. L. [1968a], A hierarchy of sets. II, Algebra i Logika, vol. 7, no. 4, pp. 15–47; English translation, Algebra and Logic, vol. 7, pp. 212–232.Google Scholar
Ershov, Yu. L. [1970], A hierarchy of sets. III, Algebra i Logika, vol. 9, pp. 34–51; English Translation, Algebra and Logic, vol. 9, pp. 20–31.Google Scholar
Friedberg, R. M. [1957], A criterion for completeness of degrees of unsolvability, this Journal, vol. 22, pp. 159–160.Google Scholar
Friedman, H. [1975], One hundred and two problems in mathematical logic, this Journal, vol. 40, pp. 113–129.Google Scholar
Harrington, L. [1975], On McLaughlin's conjecture, mimeographed handwritten notes.Google Scholar
Harrington, L. [1976], Arithmetically incomparable arithmetic singletons, mimeographed handwritten notes.Google Scholar
Harrington, L. and Kechris, A. [1975], A basis result for Σ30 sets of reals with an application to minimal covers, Proceedings of the American Mathematical Society, vol. 53, pp. 445–448.Google Scholar
Harrington, L. and Shore, R. A. [1981], Definable degrees and automorphisms of D, Bulletin (New Series) of the American Mathematical Society, vol. 4, pp. 97–99.CrossRefGoogle Scholar
Hinman, P. [1969], Some applications of forcing to hierarchy problems in arithmetic, Zeitschrift für Mathematische Logik und Grundlagen der Mathematik, vol. 15, pp. 341–352.CrossRefGoogle Scholar
Hodes, H. [1978], Uniform upper bounds on ideals of Turing degrees, this Journal, vol. 43, pp. 601–612.Google Scholar
[1980] Hodes, H., Jumping through the transfinite: The master code hierarchy of Turing degrees, this Journal, vol. 45, pp. 204–220.Google Scholar
Jockusch, C. G. Jr. [1973], An application of Σ40 determinacy to the degrees of unsolvability, this Journal, vol. 38, pp. 293–294.Google Scholar
Jockusch, C. G. Jr., and McLaughlin, T. G. [1969], Countable retracing functions and Π20 predicates, Pacific Journal of Mathematics, vol. 30, pp. 67–93.CrossRefGoogle Scholar
Jockusch, C. G. Jr., and Posner, D. [1978], Double jumps of minimal degrees, this Journal, vol. 43, pp. 715–724.Google Scholar
Jockusch, C. G. Jr., and Shore, R. A. [1983], Pseudo-jump operators. I: The r.e. case, Transactions of the American Mathematical Society, vol. 275, pp. 599–609.Google Scholar
Jockusch, C. G. Jr., and Shore, R. A. [1984], REA operators, r.e. degrees and minimal covers, Recursion theory (Cornell 1982; Nerode, A. and Shore, R. A., editors), Proceedings of Symposia in Pure Mathematics, vol. 42, American Mathematical Society, Providence, Rhode Island (to appear).Google Scholar
Jockusch, C. G. Jr., and Simpson, S. G. [1976], A degree theoretic definition of the ramified analytical hierarchy, Annals of Mathematical Logic, vol. 10, pp. 1–32.CrossRefGoogle Scholar
Jockusch, C. G. Jr., and Soare, R. I. [1970], Minimal covers and arithmetical sets, Proceedings of the American Mathematical Society, vol. 25, pp. 856–859.CrossRefGoogle Scholar
Kleene, S. C. and Post, E. L. [1954], The upper semi-lattice of degrees of unsolvability, Annals of Mathematics, vol. 59, pp. 379–407.CrossRefGoogle Scholar
Lerman, M. [1983], The degrees of unsolvability, Springer-Verlag, Berlin.CrossRefGoogle Scholar
MacIntyre, J. M. [1977], Transfinite extensions of Friedberg's completeness criterion, this Journal, vol. 42, pp. 1–10.Google Scholar
Manaster, A. [1971], Some contrasts between degrees and the arithmetical hierarchy, this Journal, vol. 36, pp. 301–304.Google Scholar
Mohrherr, J. [1982], Index sets and truth-table degrees in recursion theory, Ph.D. thesis, University of Illinois at Chicago Circle, Chicago, Illinois.Google Scholar
Mohrherr, J. [1984], A conjecture of Ershov for a relative hierarchy fails near O, Algebra i Logika (to appear); English translation, Algebra and Logic (to appear).Google Scholar
Nerode, A. and Shore, R. A. [1979], Second order logic and first order theories of reducibility orderings, The Kleene Symposium (Barwise, J., Keisler, J. and Kunen, K., editors), North-Holland, Amsterdam, pp. 181–200.Google Scholar
Nerode, A. and Shore, R. A., [1980], Reducibility orderings: theories, definability and automorphisms, Annals of Mathematical Logic, vol. 18, pp. 61–89.CrossRefGoogle Scholar
Odifreddi, P. [1983], On the first order theory of the arithmetic degrees, Proceedings of the American Mathematical Society, vol. 87, pp. 505–507.CrossRefGoogle Scholar
Posner, D. S. and Robinson, R. W. [1981], Degrees joining to 0′, this Journal, vol. 46, pp. 714–722.Google Scholar
Rogers, H. Jr. [1967], Theory of recursive functions and effective computability, McGraw-Hill, New York.Google Scholar
Rogers, H. [1967a], Some problems of definability in recursive function theory, Sets, models and recursion theory (Proceedings of the summer school in mathematical logic and tenth logic colloquium, Leicester, August–September 1965; Crossley, J. N., editor), North-Holland, Amsterdam, pp. 183–201.Google Scholar
Sacks, G. E. [1963], On the degrees less than 0′, Annals of Mathematics, ser. 2, vol. 77, pp. 211–231.CrossRefGoogle Scholar
Sacks, G. E. [1976], Countable admissible ordinals and hyperdegrees, Advances in Mathematics, vol. 20, pp. 213–262.CrossRefGoogle Scholar
Selman, A. L. [1972], Applications of forcing to the degree theory of the arithmetic hierarchy, Proceedings of the London Mathematical Society, ser. 3, vol. 25, pp. 586–602.CrossRefGoogle Scholar
Shore, R. A. [1979], The homogeneity conjecture, Proceedings of the National Academy of Sciences of the United States of America, vol. 76, pp. 4218–4219.CrossRefGoogle ScholarPubMed
Shore, R. A. [1981], The degrees of unsolvability: Global results, Logic Year 1979–80 (Lerman, M., Schmerl, J. and Soare, R., editors), Lecture Notes in Mathematics, vol. 859, Springer-Verlag, Berlin, pp. 283–301.CrossRefGoogle Scholar
Shore, R. A. [1981a], The theory of the degrees below 0′, Journal of the London Mathematical Society, vol. 24, pp. 1–14.CrossRefGoogle Scholar
Shore, R. A. [1982], On homogeneity and definability in the first order theory of the Turing degrees, this Journal, vol. 47, pp. 8–16.Google Scholar
Shore, R. A. [1984], The structure of the degrees of unsolvability, Recursion theory (Cornell, 1982; Nerode, A. and Shore, R. A., editors), Proceedings of Symposia in Pure Mathematics, vol. 42, American Mathematical Society, Providence, Rhode Island (to appear).Google Scholar
Simpson, S. G. [1977], First order theory of the degrees of recursive unsolvability, Annals of Mathematics, ser. 2, vol. 105, pp. 121–139.CrossRefGoogle Scholar