No CrossRef data available.
Published online by Cambridge University Press: 12 March 2014
The linear logic introduced in [3] by J.-Y. Girard keeps one of the so-called structural rules of the sequent calculus: the exchange rule. In a one-sided sequent calculus this rule can be formulated as
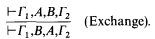
The exchange rule allows one to disregard the order of the assumptions and the order of the conclusions of a proof, and this means, when the proof corresponds to a logically correct program, to disregard the order in which the inputs and the outputs occur in a program.
In the linear logic introduced in [3], the exchange rule allows one to prove the commutativity of the multiplicative connectives, conjunction (⊗) and disjunction (⅋). Due to the presence of the exchange rule in linear logic, in the phase semantics for linear logic one starts with a commutative monoid. So, the usual linear logic may be called commutative linear logic.
The aim of the investigations underlying this paper was to see, first, what happens when we remove the exchange rule from the sequent calculus for the linear propositional logic at all, and then, how to recover the strength of the exchange rule by means of exponential connectives (in the same way as by means of the exponential connectives ! and ? we recover the strength of the weakening and contraction rules).