Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Buss, Samuel
Kołodziejczyk, Leszek
and
Zdanowski, Konrad
2015.
Collapsing modular counting in bounded arithmetic and constant depth propositional proofs.
Transactions of the American Mathematical Society,
Vol. 367,
Issue. 11,
p.
7517.
PUDLÁK, PAVEL
2017.
INCOMPLETENESS IN THE FINITE DOMAIN.
The Bulletin of Symbolic Logic,
Vol. 23,
Issue. 4,
p.
405.
Sokolov, Dmitry
2017.
Computer Science – Theory and Applications.
Vol. 10304,
Issue. ,
p.
294.
Krajíček, Jan
2018.
Randomized feasible interpolation and monotone circuits with a local oracle.
Journal of Mathematical Logic,
Vol. 18,
Issue. 02,
p.
1850012.
Lauria, Massimo
2018.
A note about k -DNF resolution.
Information Processing Letters,
Vol. 137,
Issue. ,
p.
33.
Pudlák, Pavel
and
Thapen, Neil
2019.
Random resolution refutations.
computational complexity,
Vol. 28,
Issue. 2,
p.
185.
Krajíček, Jan
2019.
Proof Complexity.
Müller, Moritz
and
Pich, Ján
2020.
Feasibly constructive proofs of succinct weak circuit lower bounds.
Annals of Pure and Applied Logic,
Vol. 171,
Issue. 2,
p.
102735.
Müller, Moritz
2021.
Typical forcings, NP search problems and an extension of a theorem of Riis.
Annals of Pure and Applied Logic,
Vol. 172,
Issue. 4,
p.
102930.
Kołodziejczyk, Leszek Aleksander
and
Thapen, Neil
2022.
Approximate counting and NP search problems.
Journal of Mathematical Logic,
Vol. 22,
Issue. 03,
Goos, Mika
Hollender, Alexandros
Jain, Siddhartha
Maystre, Gilbert
Pires, William
Robere, Robert
and
Tao, Ran
2022.
Separations in Proof Complexity and TFNP.
p.
1150.
Carmosino, Marco
Kabanets, Valentine
Kolokolova, Antonina
and
Oliveira, Igor C.
2022.
LEARN-Uniform Circuit Lower Bounds and Provability in Bounded Arithmetic.
p.
770.
Li, Jiatu
and
Oliveira, Igor C.
2023.
Unprovability of Strong Complexity Lower Bounds in Bounded Arithmetic.
p.
1051.
Ilango, Rahul
Li, Jiatu
and
Williams, R. Ryan
2023.
Indistinguishability Obfuscation, Range Avoidance, and Bounded Arithmetic.
p.
1076.
Korten, Oliver
and
Pitassi, Toniann
2024.
Strong vs. Weak Range Avoidance and the Linear Ordering Principle.
p.
1388.
Göös, Mika
Hollender, Alexandros
Jain, Siddhartha
Maystre, Gilbert
Pires, William
Robere, Robert
and
Tao, Ran
2024.
Separations in Proof Complexity and TFNP.
Journal of the ACM,
Vol. 71,
Issue. 4,
p.
1.
Chen, Lijie
Li, Jiatu
and
Oliveira, Igor C.
2024.
Reverse Mathematics of Complexity Lower Bounds.
p.
505.
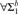 $\forall {\rm{\Sigma }}_1^b$ separations for fragments of bounded arithmetic in the relativized setting. Rather than considering the usual fragments defined by the amount of induction they allow, we study Jeřábek’s theories for approximate counting and their subtheories. We show that the
$\forall {\rm{\Sigma }}_1^b$ separations for fragments of bounded arithmetic in the relativized setting. Rather than considering the usual fragments defined by the amount of induction they allow, we study Jeřábek’s theories for approximate counting and their subtheories. We show that the  $\forall {\rm{\Sigma }}_1^b$ Herbrandized ordering principle is unprovable in a fragment of bounded arithmetic that includes the injective weak pigeonhole principle for polynomial time functions, and also in a fragment that includes the surjective weak pigeonhole principle for FPNP functions. We further give new propositional translations, in terms of random resolution refutations, for the consequences of
$\forall {\rm{\Sigma }}_1^b$ Herbrandized ordering principle is unprovable in a fragment of bounded arithmetic that includes the injective weak pigeonhole principle for polynomial time functions, and also in a fragment that includes the surjective weak pigeonhole principle for FPNP functions. We further give new propositional translations, in terms of random resolution refutations, for the consequences of  $T_2^1$ augmented with the surjective weak pigeonhole principle for polynomial time functions.
$T_2^1$ augmented with the surjective weak pigeonhole principle for polynomial time functions.
