Article contents
Jump embeddings in the Turing degrees
Published online by Cambridge University Press: 12 March 2014
Extract
Since its introduction in [K1-Po], the upper semilattice of Turing degrees has been an object of fascination to practitioners of the recursion-theoretic art. Starting from relatively simple concepts and definitions, it has turned out to be a structure of enormous complexity and richness. This paper is a contribution to the ongoing study of this structure.
Much of the work on Turing degrees may be formulated in terms of the embeddability of certain first-order structures in a structure whose universe is some set of degrees and whose relations, functions, and constants are natural degree-theoretic ones. Thus, for example, we know that if {P, ≤P) is a partial ordering of cardinality at most ℵ1 which is locally countable—each point has at most countably many predecessors—then there is an embedding
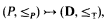
where D is the set of all Turing degrees and <T is Turing reducibility. If (P, ≤P) is a countable partial ordering, then the image of the embedding may be taken to be a subset of R, the set of recursively enumerable degrees. Without attempting to make the notion completely precise, we shall call embeddings of the first sort global, in contrast to local embeddings which impose some restrictions on the image set.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1991
References
REFERENCES
- 4
- Cited by

