Hostname: page-component-76c49bb84f-229nc Total loading time: 0 Render date: 2025-07-05T15:24:18.142Z Has data issue: false hasContentIssue false
  • English
  • Français

A note on standard systems and ultrafilters

Published online by Cambridge University Press:  12 March 2014

Fredrik Engström
Fredrik Engström*
Affiliation:
Department of Philosophy, Göteborg UniversityBox 200, 405 30 Göteborg, Sweden, E-mail: fredrik.engstrom@filosofi.gu.se
Get access Rights & Permissions [Opens in a new window]

Abstract

Let (M, 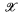)⊨ ACA0 be such that 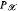, the collection of all unbounded sets in 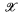, admits a definable complete ultrafilter and let T be a theory extending first order arithmetic coded in 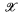such that M thinks T is consistent. We prove that there is an end-extension N ⊨ T of M such that the subsets of M coded in N are precisely those in 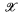. As a special case we get that any Scott set with a definable ultrafilter coding a consistent theory T extending first order arithmetic is the standard system of a recursively saturated model of T.

Information

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 73 , Issue 3 , September 2008 , pp. 824 - 830
Copyright
Copyright © Association for Symbolic Logic 2008

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

REFERENCES

[1976] Ehrenfeucht, Andrzej and Jensen, Don, Some problem in elementary arithmetics, Fundamenta Mathematical vol. 92 (1976), no. 3, pp. 223–245.Google Scholar
[2006a] Enayat, Ali, From bounded arithmetic to second order arithmetic via automorphisms, Logic in Tehran (Enayat, A., Kalantari, I., and Moniri, M., editors), Lecture Notes in Logic, vol. 26, Association for Symbolic Logic, 2006, pp. 87–113.CrossRefGoogle Scholar
[2006b] Enayat, Ali, A standard model of peano arithmetic with no conservative elementary extension, 2006.Google Scholar
[2004] Engström, Fredrik, Expansions, omitting types, and standard systems. Doctoral thesis, Chalmers University of Technology, 2004.Google Scholar
[2008] Gitman, Victoria, Scott's problem for proper scott sets, this Journal, vol. 73 (2008), no. 3, pp. 845–860.Google Scholar
[1979] Guaspari, David, Partially conservative extensions of arithmetic, Transactions of the American Mathematical Society, vol. 254 (1979), pp. 47–68.CrossRefGoogle Scholar
[2005] Hamkins, Joel and Gitman, Victoria, private communication, 2005.Google Scholar
[1991] Kaye, Richard, Models of Peano arithmetic, The Clarendon Press, Oxford University Press, New York, 1991, Oxford Science Publications.CrossRefGoogle Scholar
[1984] Kirby, Laurence, Ultrafilters and types on models of arithmetic, Annals of Pure and Applied Logic, vol. 27 (1984), no. 3, pp. 215–252.CrossRefGoogle Scholar
[1982] Knight, Julia and Nadel, Mark, Models of arithmetic and closed ideals, this Journal, vol. 47 (1982), no. 4, pp. 833–840 (1983).Google Scholar
[1962] Scott, Dana, Algebras of sets binumerable in complete extensions of arithmetic, Proceedings of symposia in pure mathematics, vol. V, American Mathematical Society, Providence, R.I., 1962, pp. 117–121.Google Scholar
[1999] Simpson, Stephen G., Subsystems of second order arithmetic, Perspectives in Mathematical Logic, Springer-Verlag, Berlin, 1999.CrossRefGoogle Scholar
[1984] Smoryński, Craig, Lectures on nonstandard models of arithmetic, Logic colloquium '82 (Florence, 1982), Studies in Logic and the Foundations of Mathematics, vol. 112, North-Holland, Amsterdam, 1984, pp. 1–70.CrossRefGoogle Scholar
[1975] Wilmers, George, Some problems in set theory; non-standard models and their applications to model theory, Ph.D. thesis, University of Oxford, 1975.Google Scholar