No CrossRef data available.
Article contents
ON REALS WITH 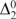 ${\rm{\Delta }}_2^0$ -BOUNDED COMPLEXITY AND COMPRESSIVE POWER
${\rm{\Delta }}_2^0$ -BOUNDED COMPLEXITY AND COMPRESSIVE POWER
Published online by Cambridge University Press: 14 September 2016
Abstract
The (prefix-free) Kolmogorov complexity of a finite binary string is the length of the shortest description of the string. This gives rise to some ‘standard’ lowness notions for reals: A is K-trivial if its initial segments have the lowest possible complexity and A is low for K if using A as an oracle does not decrease the complexity of strings by more than a constant factor. We weaken these notions by requiring the defining inequalities to hold only up to all  ${\rm{\Delta }}_2^0$ orders, and call the new notions
${\rm{\Delta }}_2^0$ orders, and call the new notions 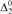 ${\rm{\Delta }}_2^0$ -bounded K-trivial and
${\rm{\Delta }}_2^0$ -bounded K-trivial and 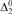 ${\rm{\Delta }}_2^0$ -bounded low for K. Several of the ‘nice’ properties of K-triviality are lost with this weakening. For instance, the new weaker definitions both give uncountable set of reals. In this paper we show that the weaker definitions are no longer equivalent, and that the
${\rm{\Delta }}_2^0$ -bounded low for K. Several of the ‘nice’ properties of K-triviality are lost with this weakening. For instance, the new weaker definitions both give uncountable set of reals. In this paper we show that the weaker definitions are no longer equivalent, and that the 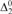 ${\rm{\Delta }}_2^0$ -bounded K-trivials are cofinal in the Turing degrees. We then compare them to other previously studied weakenings, namely infinitely-often K-triviality and weak lowness for K (in each, the defining inequality must hold up to a constant, but only for infinitely many inputs). We show that
${\rm{\Delta }}_2^0$ -bounded K-trivials are cofinal in the Turing degrees. We then compare them to other previously studied weakenings, namely infinitely-often K-triviality and weak lowness for K (in each, the defining inequality must hold up to a constant, but only for infinitely many inputs). We show that 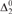 ${\rm{\Delta }}_2^0$ -bounded K-trivial implies infinitely-often K-trivial, but no implication holds between
${\rm{\Delta }}_2^0$ -bounded K-trivial implies infinitely-often K-trivial, but no implication holds between 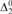 ${\rm{\Delta }}_2^0$ -bounded low for K and weakly low for K.
${\rm{\Delta }}_2^0$ -bounded low for K and weakly low for K.
Information
- Type
- Articles
- Information
- Copyright
- Copyright © The Association for Symbolic Logic 2016

