Article contents
AUTOMATIC CONTINUITY FOR ISOMETRY GROUPS
Published online by Cambridge University Press: 11 April 2017
Abstract
We present a general framework for automatic continuity results for groups of isometries of metric spaces. In particular, we prove automatic continuity property for the groups of isometries of the Urysohn space and the Urysohn sphere, i.e. that any homomorphism from either of these groups into a separable group is continuous. This answers a question of Ben Yaacov, Berenstein and Melleray. As a consequence, we get that the group of isometries of the Urysohn space has unique Polish group topology and the group of isometries of the Urysohn sphere has unique separable group topology. Moreover, as an application of our framework we obtain new proofs of the automatic continuity property for the group 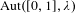 $\text{Aut}([0,1],\unicode[STIX]{x1D706})$, due to Ben Yaacov, Berenstein and Melleray and for the unitary group of the infinite-dimensional separable Hilbert space, due to Tsankov.
$\text{Aut}([0,1],\unicode[STIX]{x1D706})$, due to Ben Yaacov, Berenstein and Melleray and for the unitary group of the infinite-dimensional separable Hilbert space, due to Tsankov.
Information
- Type
- Research Article
- Information
- Copyright
- © Cambridge University Press 2017
Footnotes
The author acknowledges partial support from the following sources: the NCN (the Polish National Science Centre) grant 2012/05/D/ST1/03206, the MNiSW (the Polish Ministry of Science and Higher Education) grant 0435/IP3/2013/72 and the ‘Mobilność Plus’ program of the MNiSW through grant 630/MOB/2011/0.
References
A correction has been issued for this article:
- 9
- Cited by
Linked content
Please note a has been issued for this article.

