Hostname: page-component-cb9f654ff-hn9fh Total loading time: 0 Render date: 2025-08-11T10:20:48.790Z Has data issue: false hasContentIssue false
  • English
  • Français

DEMAZURE MODULES OF LEVEL TWO AND PRIME REPRESENTATIONS OF QUANTUM AFFINE $\mathfrak{sl}_{n+1}$

Part of: Linear algebraic groups and related topics Lie algebras and Lie superalgebras

Published online by Cambridge University Press:  09 November 2015

Matheus Brito ,
Vyjayanthi Chari  and
Adriano Moura
Matheus Brito
Affiliation:
Departamento de Matemática, Unicamp, Campinas - SP - 13083-859, Brazil (mbrito@ime.unicamp.br; aamoura@ime.unicamp.br)
Vyjayanthi Chari
Affiliation:
Department of Mathematics, University of California, Riverside, CA 92521, USA (vyjayanthi.chari@ucr.edu)
Adriano Moura
Affiliation:
Departamento de Matemática, Unicamp, Campinas - SP - 13083-859, Brazil (mbrito@ime.unicamp.br; aamoura@ime.unicamp.br)
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We study the classical limit of a family of irreducible representations of the quantum affine algebra associated to 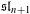$\mathfrak{sl}_{n+1}$ . After a suitable twist, the limit is a module for 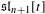$\mathfrak{sl}_{n+1}[t]$ , i.e., for the maximal standard parabolic subalgebra of the affine Lie algebra. Our first result is about the family of prime representations introduced in Hernandez and Leclerc (Duke Math. J.154 (2010), 265–341; Symmetries, Integrable Systems and Representations, Springer Proceedings in Mathematics & Statitics, Volume 40, pp. 175–193 (2013)), in the context of a monoidal categorification of cluster algebras. We show that these representations specialize (after twisting) to 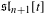$\mathfrak{sl}_{n+1}[t]$ -stable prime Demazure modules in level-two integrable highest-weight representations of the classical affine Lie algebra. It was proved in Chari et al. (arXiv:1408.4090) that a stable Demazure module is isomorphic to the fusion product of stable prime Demazure modules. Our next result proves that such a fusion product is the limit of the tensor product of the corresponding irreducible prime representations of quantum affine 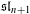$\mathfrak{sl}_{n+1}$ .

Keywords

Demazure modulesquantum affine algebrasprime representationsgraded limits

MSC classification

Primary: 17B10: Representations, algebraic theory (weights) 17B70: Graded Lie (super)algebras 20G42: Quantum groups (quantized function algebras) and their representations

Information

Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 17 , Issue 1 , February 2018 , pp. 75 - 105
Copyright
© Cambridge University Press 2015 

References

Bourbaki, N., Lie Groups and Lie Algebras IV–VI (Springer, Berlin, 2000).Google Scholar
Brito, M., Classification and structure of certain representations of quantum affine algebra, PhD thesis, Universidade Estadual de Campinas, Brazil (2015).Google Scholar
Carter, R., Llie Algebras of Finite and Affine Type (Cambridge University Press, Cambridge, 2005).Google Scholar
Chari, V., On the fermionic formula and the Kirillov–Reshetikhin conjecture, Int. Math. Res. Not. IMRN 12 (2001), 629–654.Google Scholar
Chari, V., Braid group actions and tensor products, Int. Math. Res. Not. IMRN (2002), 357–382.CrossRefGoogle Scholar
Chari, V., Fourier, G. and Khandai, T., A categorical approach to Weyl modules, Transform. Groups 15(3) (2010), 517–549.CrossRefGoogle Scholar
Chari, V. and Loktev, S., Weyl, Demazure and fusion modules for the current algebra of sl r+1 , Adv. Math. 207 (2006), 928–960.CrossRefGoogle Scholar
Chari, V. and Moura, A., The restricted Kirillov–Reshetikhin modules for the current and twisted current algebras, Comm. Math. Phys. 266(2) (2006), 431–454.CrossRefGoogle Scholar
Chari, V., Moura, A. and Young, C., Prime representations from a homological perspective, Math. Z. 274 (2013), 613–645.Google Scholar
Chari, V. and Pressley, A., Quantum affine algebras, Comm. Math. Phys. 142 (1991), 261–283.CrossRefGoogle Scholar
Chari, V. and Pressley, A., A Guide to Quantum Groups (Cambridge University Press, Cambridge, 1994).Google Scholar
Chari, V. and Pressley, A., Small representations of quantum affine algebras, Lett. Math. Phys. 30(2) (1994), 131–145.Google Scholar
Chari, V. and Pressley, A., Quantum affine algebras and their representations, in Representations of Groups (Banff, AB, 1994), CMS Conf. Proc., Volume 16, pp. 59–78 (American Mathematical Society, Providence, RI, 1995).Google Scholar
Chari, V. and Pressley, A., Minimal affinizations of representations of quantum groups: the nonsimply laced case, Lett. Math. Phys. 35 (1995), 99–114.Google Scholar
Chari, V. and Pressley, A., Quantum affine algebras and integrable quantum systems, in Quantum Fields and Quantum Space Time (Cargse, 1996), NATO Adv. Sci. Inst. Ser. B Phys., Volume 364, pp. 245–263 (Plenum, New York, 1997).Google Scholar
Chari, V., Fourier, G. and Senesi, P., Weyl modules for the twisted loop algebras, J. Algebra 319(12) (2008), 5016–5038.Google Scholar
Chari, V. and Pressley, A., Weyl modules for classical and quantum affine algebras, Represent. Theory 5 (2001), 191–223.Google Scholar
Chari, V., Shereen, P., Venkatesh, R. and Wand, J., A Steinberg type decomposition theorem for higher level Demazure modules, Preprint, 2014, arXiv:1408.4090.Google Scholar
Chari, V. and Venkatesh, R., Demazure modules, fusion products, and Q-systems, CMP (2013).Google Scholar
Demazure, M., Une nouvelle formule de caractère, Bull. Soc. Math. 98 (1974), 163–172.Google Scholar
Feigin, B. L. and Feigin, E., q-characters of the tensor products in sl2 -case, Mosc. Math. J. 2(3) (2002), 567–588. math.QA/0201111.CrossRefGoogle Scholar
Feigin, B. and Loktev, S., On generalized Kostka polynomials and the quantum verlinde rule, in Differential Topology, Infinite-Dimensional Lie Algebras, and Applications, American Mathematical Society Transl. Ser. 2, Volume 194, pp. 61–79. (1999). math.QA/9812093.Google Scholar
Fourier, G. and Littelmann, P., Tensor product structure of affine Demazure modules and limit constructions, Nagoya Math. J. 182 (2006), 171–198.Google Scholar
Fourier, G. and Littelmann, P., Weyl modules, Demazure modules, KR-modules, crystals, fusion products, and limit constructions, Adv. Math. 211(2) (2007), 566–593.Google Scholar
Frenkel, E. and Mukhin, E., Combinatorics of q-character of finite dimensional representations of quantum affine algebras, Comm. Math. Phys. 216 (2001), 23–57.Google Scholar
Hernandez, D., The Kirillov–Reshetikhin conjecture and solutions of T-systems, J. Reine Angew. Math. 2006 (2006), 63–87.Google Scholar
Hernandez, D., On minimal affinizations of representations of quantum groups, Comm. Math. Phys. 277 (2007), 221–259.Google Scholar
Hernandez, D. and Leclerc, B., Cluster algebras and quantum affine algebras, Duke Math. J. 154 (2010), 265–341. 10.1215/00127094-2010-040.Google Scholar
Hernandez, D. and Leclerc, B., Quantum Grothendieck rings and derived Hall algebras, Preprint, 2011, arXiv:1109.0862.Google Scholar
Hernandez, D. and Leclerc, B., Monoidal categorifications of cluster algebras of type A and D, in Symmetries, Integrable Systems and Representations, Springer Proceedings in Mathematics & Statistics, Volume 40, pp. 175–193 (Springer, 2013).Google Scholar
Joseph, A., On the Demazure character formula, Ann. Sci. Éc. Norm. Supér. (4) 4 (1985), 389–419.CrossRefGoogle Scholar
Kac, V., Infinite Dimensional Lie Algebras (Cambridge University Press, Cambridge, 1983).Google Scholar
Kedem, R., A pentagon of identities, graded tensor products, and the Kirillov–Reshetikhin conjecture, in New Trends in Quantum Integrable Systems, pp. 173–193 (World Science Publications, Hackensack, NJ, 2011).Google Scholar
Kumar, S., Demazure character formula in arbitrary Kac–Moody setting, Invent. Math. 89 (1987), 395–423.Google Scholar
Kumar, S., Kac–Moody groups, their Flag Varieties and Representation Theory, Progress in Mathematics (Birkhäuser Verlag, Boston, 2002).Google Scholar
Lakshmibai, V., Littelmann, P. and Magyar, P., Standard monomial theory for Bott–Samelson varieties, Compos. Math. 130 (2002), 293–318.Google Scholar
Lusztig, G., Introduction to Quantum Groups, Progress in Mathematics, vol. 110 (Birkhäuser Verlag, Boston, 1993).Google Scholar
Mathieu, O., Formules de caractères pour les algèbres de Kac–Moody générales, Astérisque, Invent. Math. (1988), 159–160.Google Scholar
Moura, A., Restricted limits of minimal affinizations, Pacific J. Math. 244 (2010), 359–397.Google Scholar
Moura, A. and Pereira, F., Graded limits of minimal affinizations and beyond: the multiplicity free case for type E 6 , Algebra Discrete Math. 12 (2011), 69–115.Google Scholar
Mukhin, E. and Young, C. A. S., Path descriptions of type B q-characters, Adv. Math. 231(2) (2012), 1119–1150.Google Scholar
Nakajima, H., t-analogs of q-characters of Kirillov–Reshetikhin modules of quantum affine algebras, Represent. Theory 7 (2003), 259–274.Google Scholar
Nakajima, H., Quiver varieties and cluster algebras, Kyoto J. Math. 51 (2011), 71–126.Google Scholar
Naoi, K., Weyl modules, Demazure modules and finite crystals for non-simply laced type, Adv. Math. 229(2) (2012), 875–934.Google Scholar
Naoi, K., Demazure modules and graded limits of minimal affinizations, Represent. Theory 17 (2013), 524–556.Google Scholar
Naoi, K., Graded limits of minimal affinizations in type D, SIGMA 10 (2014), 047, 20 pages.Google Scholar
Polo, P., Variété de Schubert et excellentes filtrations, Orbites unipotentes et représentatoins. III, Astérique (1989), 173–174, 281–311.Google Scholar
Rajan, C. S., Unique decomposition of tensor products of irreducible representations of simple algebraic groups, Ann. of Math. (2) 160(2) (2004), 683–704.Google Scholar
Ravinder, B., Demazure modules, Chari–Venkatesh modules and fusion products, SIGMA 10 (2014), 110, 10 pages.Google Scholar