Article contents
ORBIFOLD POINTS ON PRYM–TEICHMÜLLER CURVES IN GENUS  $4$
$4$
Published online by Cambridge University Press: 22 May 2017
Abstract
For each discriminant 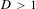 $D>1$, McMullen constructed the Prym–Teichmüller curves
$D>1$, McMullen constructed the Prym–Teichmüller curves 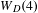 $W_{D}(4)$ and
$W_{D}(4)$ and  $W_{D}(6)$ in
$W_{D}(6)$ in  ${\mathcal{M}}_{3}$ and
${\mathcal{M}}_{3}$ and 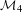 ${\mathcal{M}}_{4}$, which constitute one of the few known infinite families of geometrically primitive Teichmüller curves. In the present paper, we determine for each
${\mathcal{M}}_{4}$, which constitute one of the few known infinite families of geometrically primitive Teichmüller curves. In the present paper, we determine for each 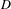 $D$ the number and type of orbifold points on
$D$ the number and type of orbifold points on 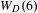 $W_{D}(6)$. These results, together with a previous result of the two authors in the genus
$W_{D}(6)$. These results, together with a previous result of the two authors in the genus  $3$ case and with results of Lanneau–Nguyen and Möller, complete the topological characterisation of all Prym–Teichmüller curves and determine their genus. The study of orbifold points relies on the analysis of intersections of
$3$ case and with results of Lanneau–Nguyen and Möller, complete the topological characterisation of all Prym–Teichmüller curves and determine their genus. The study of orbifold points relies on the analysis of intersections of 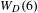 $W_{D}(6)$ with certain families of genus
$W_{D}(6)$ with certain families of genus  $4$ curves with extra automorphisms. As a side product of this study, we give an explicit construction of such families and describe their Prym–Torelli images, which turn out to be isomorphic to certain products of elliptic curves. We also give a geometric description of the flat surfaces associated to these families and describe the asymptotics of the genus of
$4$ curves with extra automorphisms. As a side product of this study, we give an explicit construction of such families and describe their Prym–Torelli images, which turn out to be isomorphic to certain products of elliptic curves. We also give a geometric description of the flat surfaces associated to these families and describe the asymptotics of the genus of 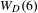 $W_{D}(6)$ for large
$W_{D}(6)$ for large  $D$.
$D$.
Information
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 18 , Issue 4 , July 2019 , pp. 673 - 706
- Copyright
- © Cambridge University Press 2017
References
- 1
- Cited by

