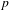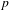Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Diamond, Fred
2024.
Compactifications of Iwahori-level Hilbert modular varieties.
Journal of Number Theory,
Vol. 263,
Issue. ,
p.
255.
Deo, Shaunak V.
Dimitrov, Mladen
and
Wiese, Gabor
2024.
Unramifiedness of weight 1 Hilbert Hecke algebras.
Algebra & Number Theory,
Vol. 18,
Issue. 8,
p.
1465.
Diamond, Fred
2025.
Kodaira–Spencer isomorphisms and degeneracy maps on Iwahori-level Hilbert modular varieties: the saving trace.
Forum of Mathematics, Sigma,
Vol. 13,
Issue. ,