Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Gitik, Moti
1986.
On precipitousness of the nonstationary ideal over a supercompact.
Journal of Symbolic Logic,
Vol. 51,
Issue. 3,
p.
648.
Gitik, Moti
1986.
Changing cofinalities and the nonstationary ideal.
Israel Journal of Mathematics,
Vol. 56,
Issue. 3,
p.
280.
Sureson, Claude
1986.
ω1-constructible universe and measurable cardinals.
Annals of Pure and Applied Logic,
Vol. 30,
Issue. 3,
p.
293.
Mitchell, W.
1987.
Applications of the covering lemma for sequences of measures.
Transactions of the American Mathematical Society,
Vol. 299,
Issue. 1,
p.
41.
Mitchell, William
1987.
Prikry forcing at κ+ and beyond.
The Journal of Symbolic Logic,
Vol. 52,
Issue. 1,
p.
44.
Drake, F. R.
1987.
European Summer Meeting of the Association for Symbolic Logic, Hull, 1986.
Journal of Symbolic Logic,
Vol. 52,
Issue. 4,
p.
1057.
Koepke, Peter
1988.
Some applications of short core models.
Annals of Pure and Applied Logic,
Vol. 37,
Issue. 2,
p.
179.
Gitik, Moti
1988.
On the Mitchell and Rudin-Kiesler orderings of ultrafilters.
Annals of Pure and Applied Logic,
Vol. 39,
Issue. 2,
p.
175.
Sureson, Claude
1989.
Chang's model and covering properties.
Annals of Pure and Applied Logic,
Vol. 42,
Issue. 1,
p.
45.
Gitik, Moti
1989.
The negation of the singular cardinal hypothesis from o(K)=K++.
Annals of Pure and Applied Logic,
Vol. 43,
Issue. 3,
p.
209.
Gitik, Moti
1989.
On generic elementary embeddings.
Journal of Symbolic Logic,
Vol. 54,
Issue. 3,
p.
700.
1989.
Logic Colloquium'87, Proceedings of the Colloquium held in Granada.
Vol. 129,
Issue. ,
p.
137.
1990.
Almost Free Modules - Set-theoretic Methods.
Vol. 46,
Issue. ,
p.
456.
Mitchell, William J.
1991.
Definable singularity.
Transactions of the American Mathematical Society,
Vol. 327,
Issue. 1,
p.
407.
Gitik, Moti
1991.
The strenght of the failure of the singular cardinal hypothesis.
Annals of Pure and Applied Logic,
Vol. 51,
Issue. 3,
p.
215.
Mitchell, William J.
1992.
Set Theory of the Continuum.
Vol. 26,
Issue. ,
p.
311.
Apter, Arthur W.
1992.
Some new upper bounds in consistency strength for certain choiceless large cardinal patterns.
Archive for Mathematical Logic,
Vol. 31,
Issue. 3,
p.
201.
Mitchell, William J.
1992.
An infinitary Ramsey property.
Annals of Pure and Applied Logic,
Vol. 57,
Issue. 2,
p.
151.
Gitik, Moti
and
Magidor, Menachem
1992.
Set Theory of the Continuum.
Vol. 26,
Issue. ,
p.
243.
Mitchell, W. J.
1992.
On the singular cardinal hypothesis.
Transactions of the American Mathematical Society,
Vol. 329,
Issue. 2,
p.
507.

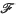 ) presented in this paper is a new inner model of
) presented in this paper is a new inner model of 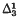 on the reals. The model
on the reals. The model 