No CrossRef data available.
Published online by Cambridge University Press: 24 October 2008
The homeotopy group Λx of a space X is the group of all homeomorphisms of X to itself, modulo the subgroup of those homeomorphisms that are isotopic to the identity. In this paper X will be taken to be a closed oriented 2-manifold, together with a polyhedral structure, and the definition of Λx is then restricted to the consideration of piecewise-linear homeomorphisms and isotopies. Although this restriction to the polyhedral category is not really essential to what follows, it does tend to simplify some of the arguments. In (2) a homeomorphism of X was associated with every simple closed (polyhedral) curve c in X in the following way. First, let A be an annulus in the Euclidean plane parametrized by (r, θ) where 1 ≤ r ≤ 2 and θ is a real number mod 2 π. We define a homeomorphism H: A → A by
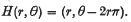
H is then fixed on the boundary of A. If now e: A → X is an orientation-preserving embedding, and eA is a neighbourhood of c in X, then eHe−1|eA can be extended by the identity on X − eA to a homeomorphism h:X → X. Any piecewise linear homeomorphism hc which is isotopic to h will be called a twist about c or, if c is not specified, just a twist.