Hostname: page-component-76fb5796d-25wd4 Total loading time: 0 Render date: 2024-04-26T07:18:54.431Z Has data issue: false hasContentIssue false
  • English
  • Français

Spirals of Riemann’s Zeta-Function — Curvature, Denseness and Universality

Part of: Zeta and $L$-functions: analytic theory

Published online by Cambridge University Press:  05 October 2023

ATHANASIOS SOURMELIDIS  and
JÖRN STEUDING
ATHANASIOS SOURMELIDIS
Affiliation:
Graz University of Technology, Steyrergasse 30, 8010 Graz, Austria. e-mail: sourmelidis@math.tugraz.at
JÖRN STEUDING
Affiliation:
University of Würzburg, Emil-Fischer-Str. 40, 97074 Würzburg, Germany. e-mail: joern.steuding@uni-wuerzburg.de
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper deals with applications of Voronin’s universality theorem for the Riemann zeta-function 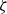$\zeta$. Among other results we prove that every plane smooth curve appears up to a small error in the curve generated by the values 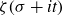$\zeta(\sigma+it)$ for real t where $\sigma\in(1/2,1)$ is fixed. In this sense, the values of the zeta-function on any such vertical line provides an atlas for plane curves. In the same framework, we study the curvature of curves generated from 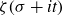$\zeta(\sigma+it)$ when 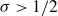$\sigma>1/2$ and we show that there is a connection with the zeros of $\zeta'(\sigma+it)$. Moreover, we clarify under which conditions the real and the imaginary part of the zeta-function are jointly universal.

MSC classification

Primary: 11M06: $zeta (s)$ and $L(s, chi)$
Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 176 , Issue 2 , March 2024 , pp. 325 - 338
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of Cambridge Philosophical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

†

Supported by the Austrian Science Fund (FWF) project number M 3246-N.

References

Andersson, J.. Lavrent’ev’s approximation theorem with nonvanishing polynomials and universality of zeta-functions. New Directions in Value-Distribution theory of Zeta and L-Functions. (Shaker Verlag, Aachen, 2009), 7–10.Google Scholar
Bagchi, B.. The statistical behaviour and universality properties of the Riemann zeta-function and other allied Dirichlet series Ph.D. Thesis. Calcutta, Indian Statistical Institute (1981).Google Scholar
Bohr, H. and Courant, R.. Neue Anwendungen der Theorie der Diophantischen Approximation auf die Riemannsche Zetafunktion. J. Reine Angew. Math. 144 (1914), 249–274.CrossRefGoogle Scholar
Borchsenius, V. and Jessen, B.. Mean motions and values of the Riemann zeta function. Acta Math. 80 (1948), 97–166.CrossRefGoogle Scholar
do Carmo, M.P.. Differential Geometry of Curves and Surfaces (Prentice-Hall, 1976).Google Scholar
Edwards, H.M.. Riemann’s Zeta Function (Academic Press, New York-London, 1974).Google Scholar
Fujii, A.. On a conjecture of Shanks. Proc. Japan Acad. Ser. A 70 (4) (1994), 109–114.CrossRefGoogle Scholar
Garunkštis, R., Laurinčikas, A., Matsumoto, K., Steuding, J. and Steuding, R.. Effective uniform approximation by the Riemann zeta-function. Publ. Mat. 54 (2010), 209–219.Google Scholar
Garunkštis, R. and Steuding, J.. On the roots of the equation $\zeta(s)=\alpha$ . Abh. Math. Seminar Univ. Hamburg 84 (2014), 1–15.CrossRefGoogle Scholar
Gonek, S.M.. Analytic Properties of Zeta and L-Functions (Ph.D. Thesis). University of Michigan, 1979.Google Scholar
Gonek, S.M. and Montgomery, H.L.. Spirals of the Zeta Function I. Analytic Number Theory. In Honour of Helmut Maier’s 60th Birthday. C. Pomerance and M.Th. Rassias (eds.) (Springer, 2015), 127–131.CrossRefGoogle Scholar
Guo, C.R., On the zeros of the derivative of the Riemann zeta function. Proc. London Math. Soc. (3) 72 (1) (1996), 28–62.CrossRefGoogle Scholar
Hartman, P.. Mean motions and almost periodic functions. Amer. Math. Soc. 46, (1939), 66–81.CrossRefGoogle Scholar
Herichi, H. and Lapidus, M.L.. Quantized Number Theory, Fractal Strings and Riemann Hypothesis (World Scientific, 2021).CrossRefGoogle Scholar
Jessen, B.. Über die Nullstellen einer analytischen fastperiodischen Funktion. Eine Verallgemeinerung der Jensenschen Formel. Math. Ann. 108 (1) (1933), 485–516.CrossRefGoogle Scholar
Jessen, B. and Tornehave, H.. Mean motions and zeros of almost periodic functions. Acta Math. 77 (1945), 137–279.CrossRefGoogle Scholar
Kalpokas, J. and Steuding, J.. On the value-distribution of the Riemann zeta-function on the critical line. Moscow J. Comb. Number Theory 1 (2011), 26–42.Google Scholar
Karatsuba, A.A. and Voronin, S.M.. The Riemann Zeta-Function (de Gruyter, 1992).CrossRefGoogle Scholar
Lapidus, M.L.. Towards quantized number theory: spectral operators and an asymmetric criterion for the Riemann hypothesis. Philos. Trans. Royal Soc., Ser. A 373 (2015), 24 pp.CrossRefGoogle Scholar
Levinson, N. and Montgomery, H.L.. Zeros of the derivatives of the Riemann zeta-function. Acta Math. 133 (1974), 49–65.CrossRefGoogle Scholar
Mergelyan, S.N.. Uniform approximations to functions of a complex variable. Usp. Mat. Nauk 7 (1952), 31-122 (Russian); Amer. Math. Soc. 101 (1954).Google Scholar
Sagan, H.. Space-Filling Curves (Springer 1994).CrossRefGoogle Scholar
Shanks, D.. Review of Haselgrove. Math. Comput. 15 (1961), 84–86.Google Scholar
Speiser, A.. Geometrisches zur Riemannschen Zetafunktion. Math. Ann. 110 (1935), 514–521.CrossRefGoogle Scholar
Sourmelidis, A. and Steuding, J.. An atlas for all plane curves. Eur. Math. Soc. Mag. (2023).CrossRefGoogle Scholar
Steuding, J.. Value-Distribution of L-Functions. Lecture Notes in Math. 1877 (Springer 2007).Google Scholar
Titchmarsh, E. C.. The Theory of the Riemann Zeta-Function (Oxford University Press, 2nd ed. revised by D.R. Heath-Brown, 1986).Google Scholar
Voronin, S.M.. The distribution of the nonzero values of the Riemann $\zeta$ -function (Russian). Collection of articles. Part II. Trudy Mat. Inst. Steklov 128 (1972), 131–150.Google Scholar
Voronin, S.M.. Theorem on the ‘universality’ of the Riemann zeta-function. Izv. Akad. Nauk SSSR, Ser. Matem. 39 (1975), 475-486 (Russian); Math. USSR Izv. 9 (1975), 443–445.Google Scholar
Voronin, S.M.. $\Omega$ -theorems of the theory of the Riemann zeta-function (Russian). Izv. Akad. Nauk SSSR Ser. Mat. 52 (1988), 424–436, 448; Math. USSR-Izv. 32 (1989), 429–442.Google Scholar
Wegert, E.. Visual Complex Functions (Birkhäuser, 2012).CrossRefGoogle Scholar
Wegert, E. and Semmler, G.. Phase plots of complex functions: a journey in illustration. Notices A.M.S. 58 (2011), 768–780.Google Scholar
Yildirim, C.. A note on $\zeta''(s)$ and $\zeta'''(s)$ . Proc. Amer. Math. Soc. 124 (1996), 2311–2314.Google Scholar