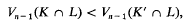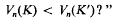Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
McMullen, P.
1977.
Use of Mathematical Literature.
p.
189.
Fáry, I.
Makai, E.
and
Pálfy, P. P.
1983.
Research problems.
Periodica Mathematica Hungarica,
Vol. 14,
Issue. 1,
p.
111.
Gruber, Peter M.
1983.
Seven small pearls from convexity.
The Mathematical Intelligencer,
Vol. 5,
Issue. 1,
p.
16.
Tanno, Shukichi
1987.
Central sections of centrally symmetric convex bodies.
Kodai Mathematical Journal,
Vol. 10,
Issue. 3,
Ball, Keith
1988.
Geometric Aspects of Functional Analysis.
Vol. 1317,
Issue. ,
p.
224.
Lutwak, Erwin
1988.
Intersection bodies and dual mixed volumes.
Advances in Mathematics,
Vol. 71,
Issue. 2,
p.
232.
Milman, V. D.
and
Pajor, A.
1989.
Geometric Aspects of Functional Analysis.
Vol. 1376,
Issue. ,
p.
64.
Giannopoulos, Apostolos A.
1990.
A note on a problem of H. Busemann and C. M. Petty concerning sections of symmetric convex bodies.
Mathematika,
Vol. 37,
Issue. 2,
p.
239.
Ball, Keith
1991.
Shadows of convex bodies.
Transactions of the American Mathematical Society,
Vol. 327,
Issue. 2,
p.
891.
Chakerian, Don
and
Logothetti, Dave
1991.
Cube Slices, Pictorial Triangles, and Probability.
Mathematics Magazine,
Vol. 64,
Issue. 4,
p.
219.
Bourgain, J.
1991.
On the Busemann-Petty problem for perturbations of the ball.
Geometric and Functional Analysis,
Vol. 1,
Issue. 1,
p.
1.
Papadimitrakis, Michael
1992.
On the Busemann‐Petty problem about convex, centrally symmetric bodies in.
Mathematika,
Vol. 39,
Issue. 2,
p.
258.
LUTWAK, Erwin
1993.
Handbook of Convex Geometry.
p.
151.
GOODEY, Paul
and
WEIL, Wolfgang
1993.
Handbook of Convex Geometry.
p.
1297.
Gardner, R. J.
1994.
Intersection bodies and the Busemann-Petty problem.
Transactions of the American Mathematical Society,
Vol. 342,
Issue. 1,
p.
435.
Koldobsky, Alexander
1997.
Intersection bodies and the Busemann-Petty problem.
Comptes Rendus de l'Académie des Sciences - Series I - Mathematics,
Vol. 325,
Issue. 11,
p.
1181.
Koldobsky, Alexander
1998.
Intersection Bodies in R4.
Advances in Mathematics,
Vol. 136,
Issue. 1,
p.
1.
Koldobsky, Alexander
1998.
Second Derivative Test for Intersection Bodies.
Advances in Mathematics,
Vol. 136,
Issue. 1,
p.
15.
Campi, Stefano
1999.
Convex intersection bodies in three and four dimensions.
Mathematika,
Vol. 46,
Issue. 1,
p.
15.
Koldobsky, A.
1999.
A generalization of the Busemann-Petty problem on sections of convex bodies.
Israel Journal of Mathematics,
Vol. 110,
Issue. 1,
p.
75.