Published online by Cambridge University Press: 02 April 2019
The relationship between interpolation and separation properties of hypersurfaces in Bargmann–Fock spaces over 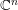 $\mathbb{C}^{n}$ is not well understood except for
$\mathbb{C}^{n}$ is not well understood except for 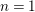 $n=1$. We present four examples of smooth affine algebraic hypersurfaces that are not uniformly flat, and show that exactly two of them are interpolating.
$n=1$. We present four examples of smooth affine algebraic hypersurfaces that are not uniformly flat, and show that exactly two of them are interpolating.
Vamsi Pritham Pingali is partially supported by the Young Investigator Award and by grant F.510/25/CAS-II/2018(SAP-I) from UGC (Govt. of India).
Please note a has been issued for this article.