Article contents
EQUIVARIANT 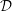 ${\mathcal{D}}$-MODULES ON ALTERNATING SENARY 3-TENSORS
${\mathcal{D}}$-MODULES ON ALTERNATING SENARY 3-TENSORS
Published online by Cambridge University Press: 29 November 2019
Abstract
We consider the space 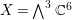 $X=\bigwedge ^{3}\mathbb{C}^{6}$ of alternating senary 3-tensors, equipped with the natural action of the group
$X=\bigwedge ^{3}\mathbb{C}^{6}$ of alternating senary 3-tensors, equipped with the natural action of the group 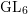 $\operatorname{GL}_{6}$ of invertible linear transformations of
$\operatorname{GL}_{6}$ of invertible linear transformations of 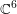 $\mathbb{C}^{6}$. We describe explicitly the category of
$\mathbb{C}^{6}$. We describe explicitly the category of 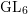 $\operatorname{GL}_{6}$-equivariant coherent
$\operatorname{GL}_{6}$-equivariant coherent  ${\mathcal{D}}_{X}$-modules as the category of representations of a quiver with relations, which has finite representation type. We give a construction of the six simple equivariant
${\mathcal{D}}_{X}$-modules as the category of representations of a quiver with relations, which has finite representation type. We give a construction of the six simple equivariant  ${\mathcal{D}}_{X}$-modules and give formulas for the characters of their underlying
${\mathcal{D}}_{X}$-modules and give formulas for the characters of their underlying  $\operatorname{GL}_{6}$-structures. We describe the (iterated) local cohomology groups with supports given by orbit closures, determining, in particular, the Lyubeznik numbers associated to the orbit closures.
$\operatorname{GL}_{6}$-structures. We describe the (iterated) local cohomology groups with supports given by orbit closures, determining, in particular, the Lyubeznik numbers associated to the orbit closures.
Information
- Type
- Article
- Information
- Copyright
- © 2019 Foundation Nagoya Mathematical Journal
References
- 4
- Cited by


