Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Arai, Keisuke
and
Momose, Fumiyuki
2010.
Rational points on X0+(37M).
Journal of Number Theory,
Vol. 130,
Issue. 10,
p.
2272.
Rouse, Jeremy
Sutherland, Andrew V.
and
Zureick-Brown, David
2022.
-adic images of Galois for elliptic curves over (and an appendix with John Voight).
Forum of Mathematics, Sigma,
Vol. 10,
Issue. ,

 (= the maximal unramified extension of
(= the maximal unramified extension of 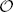 its integer ring,
its integer ring,  .
.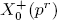 . (This criterion is Momose’s theorem in [14] except for the cases
. (This criterion is Momose’s theorem in [14] except for the cases 