No CrossRef data available.
Article contents
ON THE STANDARD TWIST OF THE 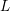 $L$-FUNCTIONS OF HALF-INTEGRAL WEIGHT CUSP FORMS
$L$-FUNCTIONS OF HALF-INTEGRAL WEIGHT CUSP FORMS
Published online by Cambridge University Press: 26 December 2018
Abstract
The standard twist 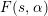 $F(s,\unicode[STIX]{x1D6FC})$ of
$F(s,\unicode[STIX]{x1D6FC})$ of 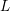 $L$-functions
$L$-functions 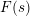 $F(s)$ in the Selberg class has several interesting properties and plays a central role in the Selberg class theory. It is therefore natural to study its finer analytic properties, for example the functional equation. Here we deal with a special case, where
$F(s)$ in the Selberg class has several interesting properties and plays a central role in the Selberg class theory. It is therefore natural to study its finer analytic properties, for example the functional equation. Here we deal with a special case, where  $F(s)$ satisfies a functional equation with the same
$F(s)$ satisfies a functional equation with the same 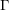 $\unicode[STIX]{x1D6E4}$-factor of the
$\unicode[STIX]{x1D6E4}$-factor of the 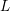 $L$-functions associated with the cusp forms of half-integral weight; for simplicity we present our results directly for such
$L$-functions associated with the cusp forms of half-integral weight; for simplicity we present our results directly for such 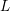 $L$-functions. We show that the standard twist
$L$-functions. We show that the standard twist 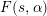 $F(s,\unicode[STIX]{x1D6FC})$ satisfies a functional equation reflecting
$F(s,\unicode[STIX]{x1D6FC})$ satisfies a functional equation reflecting  $s$ to
$s$ to 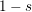 $1-s$, whose shape is not far from a Riemann-type functional equation of degree 2 and may be regarded as a degree 2 analog of the Hurwitz–Lerch functional equation. We also deduce some results on the growth on vertical strips and on the distribution of zeros of
$1-s$, whose shape is not far from a Riemann-type functional equation of degree 2 and may be regarded as a degree 2 analog of the Hurwitz–Lerch functional equation. We also deduce some results on the growth on vertical strips and on the distribution of zeros of 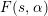 $F(s,\unicode[STIX]{x1D6FC})$.
$F(s,\unicode[STIX]{x1D6FC})$.
Information
- Type
- Article
- Information
- Copyright
- © 2018 Foundation Nagoya Mathematical Journal


