Published online by Cambridge University Press: 20 January 2009
The classical theorem of Müntz and Szász says that the span of
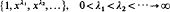
is dense in C[0,1] in the uniform norm if and only if 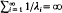 . We prove that, if {λi} is lacunary, we can replace the underlying interval [0,1] by any set of positive measure. The key to the proof is the establishment of a bounded Remez-type inequality for lacunary Müntz systems. Namely if A ⊆ [0,1] and its Lebesgue measure µ(A) is at least ε > 0 then
. We prove that, if {λi} is lacunary, we can replace the underlying interval [0,1] by any set of positive measure. The key to the proof is the establishment of a bounded Remez-type inequality for lacunary Müntz systems. Namely if A ⊆ [0,1] and its Lebesgue measure µ(A) is at least ε > 0 then
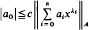
where c depends only on ε and Λ (not on n and A) and where Λ:=infiλi+1/λi>1.