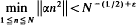Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Baker, R. C.
1983.
Small solutions of congruences.
Mathematika,
Vol. 30,
Issue. 2,
p.
164.
Vaaler, Jeffrey D.
1985.
Some extremal functions in Fourier analysis.
Bulletin of the American Mathematical Society,
Vol. 12,
Issue. 2,
p.
183.
1987.
Geometry of Numbers.
Vol. 37,
Issue. ,
p.
632.
Heath-Brown, D. R.
1991.
Small solutions of quadratic congruences, II.
Mathematika,
Vol. 38,
Issue. 2,
p.
264.
Baker, R. C.
and
Brüdern, J.
1993.
Pairs of quadratic forms modulo one.
Glasgow Mathematical Journal,
Vol. 35,
Issue. 1,
p.
51.
Baker, Roger
2023.
Diophantine approximation with smooth numbers.
The Ramanujan Journal,
Vol. 61,
Issue. 1,
p.
49.