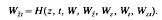Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Dai, Dao-qing
1994.
The Riemann-Hilbert boundary value problem for semilinear pseudoparabolic equations.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 23,
Issue. 6,
p.
785.
Dai, D. Q.
1999.
Complex Methods for Partial Differential Equations.
Vol. 6,
Issue. ,
p.
21.
Begehr, Heinrich
and
Dai, Dao-Qing
2004.
On continuous solutions of a generalized Cauchy–Riemann system with more than one singularity.
Journal of Differential Equations,
Vol. 196,
Issue. 1,
p.
67.
Dai, Dao-Qing
and
Liu, Ming-Sheng
2005.
Spectrum of the Riemann–Hilbert–Poincaré problem for analytic functions.
Complex Variables, Theory and Application: An International Journal,
Vol. 50,
Issue. 7-11,
p.
497.
Dai, Dao-Qing
and
Huang, Yu
2006.
On a nonlocal problem for nonlinear pseudoparabolic equations.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 64,
Issue. 3,
p.
499.
Dai, Dao-Qing
and
Huang, Yu
2007.
A moment problem for one-dimensional nonlinear pseudoparabolic equation.
Journal of Mathematical Analysis and Applications,
Vol. 328,
Issue. 2,
p.
1057.
Dai, Dao-Qing
and
Huang, Yu
2007.
Nonlocal boundary problems for a third-order one-dimensional nonlinear pseudoparabolic equation.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 66,
Issue. 1,
p.
179.
Yang, Cao
and
Jingxue, Yin
2024.
An overview of recent studies on the pseudo-parabolic equation.
SCIENTIA SINICA Mathematica,
Vol. 54,
Issue. 3,
p.
259.