Published online by Cambridge University Press: 14 November 2011
We study the system 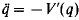 in RN, where V is a potential with a strict local maximum at 0 and possibly with a singularity. First, using a minimising argument, we can prove the existence of a homoclinic orbit when the component Ω of {x ∈ RN: V(x) < V(0)} containing 0 is an arbitrary open set; in the case Ω unbounded we allow V(x) to go to 0 at infinity, although at a slow enough rate. Then we show that the presence of a singularity in Ω implies that a homoclinic solution can also be found via a minimax procedure and, comparing the critical levels of the functional associated to the system, we see that the two solutions are distinct whenever the singularity is ‘not too far’ from 0.
in RN, where V is a potential with a strict local maximum at 0 and possibly with a singularity. First, using a minimising argument, we can prove the existence of a homoclinic orbit when the component Ω of {x ∈ RN: V(x) < V(0)} containing 0 is an arbitrary open set; in the case Ω unbounded we allow V(x) to go to 0 at infinity, although at a slow enough rate. Then we show that the presence of a singularity in Ω implies that a homoclinic solution can also be found via a minimax procedure and, comparing the critical levels of the functional associated to the system, we see that the two solutions are distinct whenever the singularity is ‘not too far’ from 0.