Hostname: page-component-76fb5796d-25wd4 Total loading time: 0 Render date: 2024-04-28T03:57:50.864Z Has data issue: false hasContentIssue false
  • English
  • Français

Limit cycles in a rotated family of generalized Liénard systems allowing for finitely many switching lines

Part of: Qualitative theory

Published online by Cambridge University Press:  21 March 2024

Hebai Chen Open the ORCID record for Hebai Chen [Opens in a new window] ,
Yilei Tang Open the ORCID record for Yilei Tang [Opens in a new window]  and
Weinian Zhang
Hebai Chen
Affiliation:
School of Mathematics and Statistics, HNP-LAMA, Central South University, Changsha, Hunan 410083, P. R. China (chen_hebai@csu.edu.cn)
Yilei Tang
Affiliation:
School of Mathematical Sciences, CMA-Shanghai, Shanghai Jiao Tong University, Shanghai 200240, P. R. China (mathtyl@sjtu.edu.cn)
Weinian Zhang
Affiliation:
Department of Mathematics, Sichuan University, Chengdu, Sichuan 610064, P. R. China (wnzhang@scu.edu.cn)
Get access Rights & Permissions [Opens in a new window]

Abstract

Analytic rotated vector fields have four significant properties: as the rotated parameter $\alpha$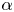 changes, the amplitude of each stable (or unstable) limit cycle varies monotonically, each semi-stable limit cycle bifurcates at most two limit cycles, the isolated homoclinic loop (if exists) disappears while a unique limit cycle with the same stability arises or no closed orbits arise oppositely, and a unique limit cycle arises near the weak focus (if exists). In this paper, we prove that the four properties remain true for a rotated family of generalized Liénard systems having finitely many switching lines. Furthermore, we discuss variational exponent and use it to formulate multiplicity of limit cycles. Then we apply our results to give exact number of limit cycles to a continuous piecewise linear system with three zones and answer to a question on the maximum number of limit cycles in an SD oscillator.

Keywords

rotated vector fieldswitching generalized Liénard systemlimit cyclehomoclinic loop

MSC classification

Primary: 34C05: Location of integral curves, singular points, limit cycles
Secondary: 34C07: Theory of limit cycles of polynomial and analytic vector fields (existence, uniqueness, bounds, Hilbert's 16th problem and ramifications) 34C23: Bifurcation 34C37: Homoclinic and heteroclinic solutions
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , First View , pp. 1 - 29
Check for updates
Copyright
Copyright © The Author(s), 2024. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

di Bernardo, M., Budd, C. J., Champneys, A. R. and Kowalczyk, P.. Piecewise-Smooth Dynamical Systems: Theory and Applications (London: Springer, 2008).Google Scholar
di Bernardo, M., Budd, C. J., Champneys, A. R., Kowalczyk, P., Nordmark, A. B., Tost, G. O. and Piiroinen, P. T.. Bifurcations in nonsmooth dynamical systems. SIAM Rev 50 (2008), 629–701.CrossRefGoogle Scholar
Cândido, M. R., Llibre, J. and Valls, C.. Non-existence, existence, and uniqueness of limit cycles for a generalization of the van der Pol–Duffing and the Rayleigh–Duffing oscillators. Phys. D 407 (2020), 132458.CrossRefGoogle Scholar
Chen, H., Llibre, J. and Tang, Y.. Global study of SD oscillator. Nonlinear Dyn. 91 (2018), 1755–1777.CrossRefGoogle Scholar
Chen, H. and Tang, Y.. At most two limit cycles in a piecewise linear differential system with three zones and asymmetry. Phys. D 386–387 (2019), 23–30.CrossRefGoogle Scholar
Chow, S. N. and Hale, J. K.. Methods of Bifurcation Theory (New York: Springer, 1982).CrossRefGoogle Scholar
Chow, S. N., Li, C. and Wang, D.. Normal Forms and Bifurcation of Planar Vector Fields (London: Cambridge University Press, 1994).CrossRefGoogle Scholar
Duff, G. F. D.. Limit cycles and rotated vector fields. Ann. Math 57 (1953), 15–31.CrossRefGoogle Scholar
Dumortier, F. and Li, C.. Quadratic Liénard equations with quadratic damping. J. Differ. Equ. 139 (1997), 41–59.CrossRefGoogle Scholar
Dumortier, F. and Li, C.. Perturbations from an elliptic Hamiltonian of degree four. II. Cuspidal loop. J. Differ. Equ. 175 (2001), 209–243.CrossRefGoogle Scholar
Dumortier, F., Llibre, J. and Artés, J. C.. Qualitative Theory of Planar Differential Systems (New York: Springer, 2006).Google Scholar
Dumortier, F. and Rousseau, C.. Cubic Liénard equations with linear damping. Nonlinearity 3 (1990), 1015–1039.CrossRefGoogle Scholar
Euzébio, R., Pazim, R. and Ponce, E.. Jump bifurcations in some degenerate planar piecewise linear differential systems with three zones. Physica D 325 (2016), 74–85.CrossRefGoogle Scholar
Han, M.. Global behavior of limit cycles in rotated vector fields. J. Differ. Equ. 151 (1999), 20–35.CrossRefGoogle Scholar
Kunze, M.. Non-Smooth Dynamical Systems (Berlin: Springer, 2000).CrossRefGoogle Scholar
Kuznetsov, Yu.A., Rinaldi, S. and Gragnani, A.. One-parameter bifurcations in planar Filippov systems. Internat. J. Bifur. Chaos 13 (2003), 2157–2188.CrossRefGoogle Scholar
Llibre, J., Ordóñez, M. and Ponce, E.. On the existence and uniqueness of limit cycles in planar continuous piecewise linear systems without symmetry. Nonlinear Anal. RWA 14 (2013), 2002–2012.CrossRefGoogle Scholar
Llibre, J., Ponce, E. and Valls, C.. Uniqueness and non-uniqueness of limit cycles for piecewise linear differential systems with three zones and no symmetry. J. Nonlinear Sci. 25 (2015), 861–887.CrossRefGoogle Scholar
Llibre, J., Ponce, E. and Valls, C.. Two limit cycles in Liénard piecewise linear differential systems. J. Nonlinear Sci. 29 (2019), 1499–1522.CrossRefGoogle Scholar
Perko, L. M.. Rotated vector fields and the global behavior of limit cycles for a class of quadratic systems in the plane. J. Differ. Equ. 18 (1975), 63–86.CrossRefGoogle Scholar
Perko, L. M.. Global families of limit cycles of planar analytic systems. Trans. Amer. Math. Soc 322 (1990), 627–656.CrossRefGoogle Scholar
Perko, L. M.. A global analysis of the Bogdanov-Takens system. SIAM J. Appl. Math 52 (1992), 1172–1192.CrossRefGoogle Scholar
Perko, L. M.. Rotated vector fields. J. Differ. Equ. 103 (1993), 127–145.CrossRefGoogle Scholar
Perko, L. M.. Homoclinic loop and multiple limit cycle bifurcation surfaces. Trans. Amer. Math. Soc 344 (1994), 101–130.CrossRefGoogle Scholar
Perko, L. M.. Multiple limit cycle bifurcation surfaces and global families of multiple limit cycles. J. Differ. Equ. 122 (1995), 89–113.CrossRefGoogle Scholar
Perko, L. M.. Differential Equations and Dynamical Systems. 3rd Ed. (New York: Springer, 2002).Google Scholar
Sun, Y. and Liu, C.. The Poincaré Bifurcation of a SD Oscillator. Discrete Contin. Dyn. Syst. Ser. B 26 (2021), 1565–1577.Google Scholar
Ye, Y.. A qualitative study of the integral curves of a quadratic differential equation, $I$ and $II$. Sci. China Ser. A 3 (1963), 62–70.Google Scholar
Ye, Y.. Rotated vector fields decomposition method and its application, Bifurcations of Planar Vector Fields (Luminy, 1989), Lecture Notes in Math. Vol. 1455 (Springer, Berlin, 1990), pp. 385–392.Google Scholar
Zhang, Z., Ding, T., Huang, W. and Dong, Z.. Qualitative Theory of Differential Equations, Transl. Math. Monogr. Vol. 101 (Amer. Math. Soc., Providence, RI, 1992).Google Scholar