Hostname: page-component-84b7d79bbc-2l2gl Total loading time: 0 Render date: 2024-08-03T15:41:10.650Z Has data issue: false hasContentIssue false
  • English
  • Français

A STUDY OF TRUTH PREDICATES IN MATRIX SEMANTICS

Published online by Cambridge University Press:  08 June 2018

TOMMASO MORASCHINI
TOMMASO MORASCHINI*
Affiliation:
Institute of Computer Science, Czech Academy of Sciences
*
*DEPARTMENT OF THEORATICAL COMPUTER SCIENCE INSTITUTE OF COMPUTER SCIENCE, CZECH ACADEMY OF SCIENCES POD VODÁRENSKOU VĚŽÍ 2 182 07 PRAGUE 8, CZECH REPUBLIC E-mail: moraschini@cs.cas.cz
Get access Rights & Permissions [Opens in a new window]

Abstract

Abstract algebraic logic is a theory that provides general tools for the algebraic study of arbitrary propositional logics. According to this theory, every logic 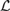${\cal L}$ is associated with a matrix semantics $Mo{d^{\rm{*}}}{\cal L}$. This article is a contribution to the systematic study of the so-called truth sets of the matrices in 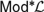$Mo{d^{\rm{*}}}{\cal L}$. In particular, we show that the fact that the truth sets of 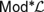$Mo{d^{\rm{*}}}{\cal L}$ can be defined by means of equations with universally quantified parameters is captured by an order-theoretic property of the Leibniz operator restricted to deductive filters of 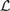${\cal L}$. This result was previously known for equational definability without parameters. Similarly, it was known that the truth sets of 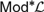$Mo{d^{\rm{*}}}{\cal L}$ are implicitly definable if and only if the Leibniz operator is injective on deductive filters of 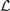${\cal L}$ over every algebra. However, it was an open problem whether the injectivity of the Leibniz operator transfers from the theories of 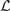${\cal L}$ to its deductive filters over arbitrary algebras. We show that this is the case for logics expressed in a countable language, and that it need not be true in general. Finally we consider an intermediate condition on the truth sets in 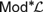$Mo{d^{\rm{*}}}{\cal L}$ that corresponds to the order-reflection of the Leibniz operator.

Keywords

03G2703G1003B22abstract algebraic logictruth predicateequational definabilitytruth-equational logicprotoalgebraic logicLeibniz hierarchyLeibniz operatorimplicit definabilitymatrix semanticsalgebraic semanticspropositional logicprotodisjunctionprotoconjunction
Type
Research Article
Information
The Review of Symbolic Logic , Volume 11 , Issue 4 , December 2018 , pp. 780 - 804
Copyright
Copyright © Association for Symbolic Logic 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

BIBLIOGRAPHY

Albuquerque, H., Font, J. M., Jansana, R., & Moraschini, T. (2018). Assertional logics, truth-equational logics, and the hierarchies of abstract algebraic logic. In Czelakowski, J., editor. Don Pigozzi on Abstract Algebraic Logic and Universal Algebra. Outstanding Contributions, Vol. 16. Berlin: Springer-Verlag, pp. 53–79.Google Scholar
Babyonyshev, S. V. (2003). Fully Fregean logics. Reports on Mathematical Logic, 37, 59–77.Google Scholar
Blok, W. J. & Pigozzi, D. (1986). Protoalgebraic logics. Studia Logica, 45, 337–369.CrossRefGoogle Scholar
Blok, W. J. & Pigozzi, D. (1989). Algebraizable Logics. Memoirs of the American Mathematical Society, Vol. 396. Providence: American Mathematical Society.Google Scholar
Blok, W. J. & Pigozzi, D. (1992). Algebraic semantics for universal Horn logic without equality. In Romanowska, A. and Smith, J. D. H., editors. Universal Algebra and Quasigroup Theory. Berlin: Heldermann, pp. 1–56.Google Scholar
Blok, W. J. & Rebagliato, J. (2003). Algebraic semantics for deductive systems. Studia Logica, Special Issue on Abstract Algebraic Logic, Part II, 74(5), 153–180.Google Scholar
Bou, F. & Rivieccio, U. (2011). The logic of distributive bilattices. Logic Journal of the Interest Group in Pure and Applied Logics, 19(1), 183–216.Google Scholar
Czelakowski, J. (1985). Algebraic aspects of deduction theorems. Studia Logica, 44, 369–387.CrossRefGoogle Scholar
Czelakowski, J. (1986). Local deductions theorems. Studia Logica, 45, 377–391.CrossRefGoogle Scholar
Czelakowski, J. (2001). Protoalgebraic Logics. Trends in Logic—Studia Logica Library, Vol. 10. Dordrecht: Kluwer Academic Publishers.Google Scholar
Czelakowski, J. (2003). The Suszko operator. Part I. Studia Logica, Special Issue on Abstract Algebraic Logic, Part II, 74(5), 181–231.Google Scholar
Czelakowski, J. & Jansana, R. (2000). Weakly algebraizable logics. The Journal of Symbolic Logic, 65(2), 641–668.CrossRefGoogle Scholar
Czelakowski, J. & Pigozzi, D. (2004). Fregean logics. Annals of Pure and Applied Logic, 127(1–3), 17–76.CrossRefGoogle Scholar
Czelakowski, J. & Pigozzi, D. (2004). Fregean logics with the multiterm deduction theorem and their algebraization. Studia Logica, 78(1–2), 171–212.CrossRefGoogle Scholar
Descalço, L. & Martins, M. A. (2005). On the injectivity of the Leibniz operator. Bulletin of the Section of Logic, 34(4), 203–211.Google Scholar
Font, J. M. (2016). Abstract Algebraic Logic - An Introductory Textbook. Studies in Logic - Mathematical Logic and Foundations, Vol. 60. London: College Publications.Google Scholar
Font, J. M., Guzmán, F., & Verdú, V. (1991). Characterization of the reduced matrices for the $\{ \wedge , \vee \}$-fragment of classical logic. Bulletin of the Section of Logic, 20, 124–128.Google Scholar
Font, J. M. & Jansana, R. (2009). A General Algebraic Semantics for Sentential Logics (second edition 2017). Lecture Notes in Logic, Vol. 7. Cambridge: Cambridge University Press.Google Scholar
Font, J. M., Jansana, R., & Pigozzi, D. (2003). A survey on abstract algebraic logic. Studia Logica, Special Issue on Abstract Algebraic Logic, Part II, 74(1–2), 13–97. With an “Update” in 91(2009), 125–130.Google Scholar
Font, J. M. & Moraschini, T. (2014). Logics of varieties, logics of semilattices, and conjunction. Logic Journal of the Interest Group in Pure and Applied Logics, 22, 818–843.Google Scholar
Font, J. M. & Moraschini, T. (2014). A note on congruences of semilattices with sectionally finite height. Algebra Universalis, 72(3), 287–293.CrossRefGoogle Scholar
Font, J. M. & Verdú, V. (1991). Algebraic logic for classical conjunction and disjunction. Studia Logica, Special Issue on Algebraic Logic, 50, 391–419.Google Scholar
Ginsberg, M. L. (1988). Multivalued logics: A uniform approach to inference in artificial intelligence. Computational Intelligence, 4, 265–316.CrossRefGoogle Scholar
Herrmann, B. (1993). Algebraizability and Beth’s theorem for equivalential logics. Bulletin of the Section of Logic, 22(2), 85–88.Google Scholar
Herrmann, B. (1993). Equivalential Logics and Definability of Truth. Ph.D. Thesis, Berlin, Freie Universität.Google Scholar
Herrmann, B. (1997). Characterizing equivalential and algebraizable logics by the Leibniz operator. Studia Logica, 58, 305–323.CrossRefGoogle Scholar
Jansana, R. & Moraschini, T. (2017). Advances in the Theory of the Leibniz Hierarchy. Manuscript.Google Scholar
Moraschini, T. (2016). Investigations in the Role of Translations in Abstract Algebraic Logic. Ph.D. Thesis, University of Barcelona.Google Scholar
Moraschini, T. (2016). On the Complexity of the Leibniz Hierarchy. Submitted manuscript.Google Scholar
Moraschini, T. (2018). A computational glimpse at the Leibniz and Frege hierarchies. Annals of Pure and Applied Logic, 169(1), 1–20.CrossRefGoogle Scholar
Noguera, C. & Cintula, P. (2013). The proof by cases property and its variants in structural consequence relations. Studia Logica, 101, 713–747.Google Scholar
Raftery, J. G. (2006). The equational definability of truth predicates. Reports on Mathematical Logic, 41, 95–149.Google Scholar
Raftery, J. G. (2011). A perspective on the algebra of logic. Quaestiones Mathematicae, 34, 275–325.CrossRefGoogle Scholar
Rautenberg, W. (1993). On reduced matrices. Studia Logica, 52, 63–72.CrossRefGoogle Scholar
Rivieccio, U. (2010). An Algebraic Study of Bilattice-based Logics. Ph.D. Dissertation, University of Barcelona.Google Scholar