Hostname: page-component-78c5997874-fbnjt Total loading time: 0 Render date: 2024-11-19T01:13:20.107Z Has data issue: false hasContentIssue false
  • English
  • Français

Functional equations and the Galton-Watson process

Published online by Cambridge University Press:  01 July 2016

E. Seneta
E. Seneta*
Affiliation:
Australian National University
Get access Rights & Permissions [Opens in a new window]

Extract

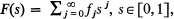In the present exposition we are concerned only with the simple Galton-Watson process, initiated by a single ancestor (Harris (1963), Chapter I). Let denote the probability generating function of the offspring distribution of a single individual. Our fundamental assumption, which holds throughout the sequel, is that fj ≠ 1, j = 0,1,2, …; in particular circumstances it shall be necessary to strengthen this to 0 < f0 ≡ F(0) < 1, which is the relevant assumption when extinction behaviour is to be considered. (Even so, our assumptions will always differ slightly from those of Harris (1963), p. 5.)

Type
Research Article
Information
Advances in Applied Probability , Volume 1 , Issue 1 , Spring 1969 , pp. 1 - 42
Copyright
Copyright © Applied Probability Trust 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bartlett, M. S. (1955) An Introduction to Stochastic Processes. Cambridge University Press, Cambridge.Google Scholar
Breny, H. (1962) Sur un point de la théorie des files d'attente. Ann. Soc. Sci. Bruxelles 76, 5–12.Google Scholar
Coifman, R. (1965) Sur l'unicité des solutions de l'équation d'Abel-Schröder et l'itéation continue. J. Aust. Math. Soc. 5, 36–47.CrossRefGoogle Scholar
Dunne, M. C. and Potts, R. B. (1965) Analysis of a computer control of an isolated intersection. Proceedings of the Third International Symposium on the Theory of Traffic Flow, New York.Google Scholar
Feller, W. (1957) An Introduction to Probability Theory and its Applications. Vol. I. Wiley, New York.Google Scholar
Gnedenko, B. V. and Kovalenko, I. N. (1966) Introduction to the Theory of Mass Service (in Russian). “Nauka”, Moscow.Google Scholar
Haldane, J. B. S. (1949) Some statistical problems arising in genetics. J. R. Statist. Soc. B 11, 1–14.Google Scholar
Harris, T. E. (1963) The Theory of Branching Processes. Springer, Berlin.CrossRefGoogle Scholar
Heathcote, C. R. (1965) A branching process allowing immigration. J. R. Statist. Soc. B 27, 138–143.Google Scholar
Heathcote, C. R. (1966) Corrections and comments on the paper “A branching process allowing immigration”. J. R. Statist. Soc. B 28, 213–217.Google Scholar
Heathcote, C. R., Seneta, E. and Vere-Jones, D. (1967) A refinement of two theorems in the theory of branching processes. Teor. Veroyat. Primen. 12, 341–346.Google Scholar
Joffe, A. (1967) On the Galton-Watson branching process with mean less than one. Ann. Math. Statist. 38, 264–266.CrossRefGoogle Scholar
Karlin, S. and McGregor, J. L. (1966) Spectral theory of branching processes. I. Z. Wahrscheinlichkeitsth. 5, 6–33.CrossRefGoogle Scholar
Kendall, D. G. (1966a) Branching processes since 1873. J. London Math. Soc. 41, 385–406.CrossRefGoogle Scholar
Kendall, D. G. (1966b) On supercritical branching processes with a positive chance of extinction. Research Papers in Statistics. Festschrift for J. Neyman, 157–165.Google Scholar
Kesten, H. (1966) (Private communication).Google Scholar
Kesten, H., Ney, P. and Spitzer, F. (1966) The Galton-Watson process with mean one and finite variance. Teor. Veroyat. Primen. 11, 579–611.Google Scholar
Kesten, H. and Stigum, B. P. (1966) A limit theorem for multidimensional Galton-Watson processes. Ann. Math. Statist. 37, 1211–1223.CrossRefGoogle Scholar
Khintchine, A. (1948) Asymptotische Gesetze der Wahrscheinlichkeitsrechnung. Chelsea, New York.Google Scholar
Kingman, J. F. C. (1961) The single server queue in heavy traffic. Proc. Camb. Phil. Soc. 57, 902–904.CrossRefGoogle Scholar
Kneser, H. (1950) Reelle analytische Lösungen der Gleichung ϕ(ϕ)(x)) = e x und ver-wandter Funktionalgleichungen. J. reine angew. Math. 187, 56–67.CrossRefGoogle Scholar
Kolmogorov, A. N. (1938) Zur Lösung einer biologischen Aufgabe. Comm. Math. Mech. Chebychev Univ., Tomsk 2, 1–6.Google Scholar
Kuczma, M. (1960) Remarques sur quelques théorèmes de J. Anastassiadis. Bull. Sci. Math. 84, 98–102.Google Scholar
Kuczma, M. (1961) Sur une équation fonctionelle. Mathematica (Cluj) 3, 79–87.Google Scholar
Kuczma, M. (1963) On the Schröder equation. Rozprawy Mat. 34.Google Scholar
Kuczma, M. (1964a) A survey of the theory of functional equations. Publikacije Elektrotechničkog Fakulteta, Univerzitet u Beogradu, Serija: Matematika i Fizika, No. 130.Google Scholar
Kuczma, M. (1964b) Note on Schröder's functional equation. J. Aust. Math. Soc. 4, 149–151.CrossRefGoogle Scholar
Kuczma, M. (1965) On convex solutions of Abel's functional equation. Bull. Acad. Polon. Sci. (Math., Astr., Phys.) 13, 645–648.Google Scholar
Kuczma, M. (1966) Sur l'équation de Böttcher. Mathematica (Cluj) 8, 279–285.Google Scholar
Kuczma, M. (1967) Un théorème de l'unicité pour l'équation fonctionelle de Böttcher. Mathematica (Cluj) (to appear).Google Scholar
Kuczma, M. (1968) Functional Equations in a Single Variable. Monografie Matematyczne Vol. 46, Warsaw.Google Scholar
Kuczma, M. and Smajdor, A. (1967) Note on iteration of concave functions. Amer. Math. Monthly 74, 401–402.CrossRefGoogle Scholar
Levinson, N. (1959) Limiting theorems for Galton-Watson branching process. Illinois J. Math. 3, 554–565.CrossRefGoogle Scholar
Levy, P. (1928) Fonctions à croissance régulière et itération d'ordre fractionnaire. Ann. Mat. Pura Applic. 5, 269–298.CrossRefGoogle Scholar
Nagaev, A. V. (1961) A refinement of certain theorems of the theory of branching random processes (in Russian). Trudy Tashkent. Univ. (im Lenin) 189, 55–63.Google Scholar
Nagaev, A. V. and Badalbaev, I. (1967) A refinement of certain theorems on branching random processes (in Russian). Litovsk. Mat. Sb. 7, 129–136.Google Scholar
Papangelou, F. (1967) A lemma on the Galton-Watson process. Z. Wahrscheinlichkeitsth. (to appear).Google Scholar
Picard, E. (1950) Leçons sur Quelques Équations Fonctionelles. Gauthier-Villars, Paris.Google Scholar
Reuter, G. E. H. (1968) (Private communication).Google Scholar
Seneta, E. (1967) The Galton-Watson process with mean one. J. Appl. Prob. 4, 489–495.CrossRefGoogle Scholar
Seneta, E. (1968a) Topics in the Theory and Applications of Markov Chains. , Australian National University.Google Scholar
Seneta, E. (1968b) The stationary distribution of a branching process allowing immigration: a remark on the critical case. J. R. Statist. Soc. B 30, 176–179.Google Scholar
Seneta, E. (1968c) On asymptotic properties of subcritical branching processes. J. Aust. Math. Soc. 8, 671–682.CrossRefGoogle Scholar
Seneta, E. (1969) On Koenigs' ratios for iterates of real functions (to appear).CrossRefGoogle Scholar
Seneta, E. and Vere-Jones, D. (1966) On quasi-stationary distributions in discrete-time Markov chains with a denumerable infinity of states. J. Appl. Prob. 3, 403–434.CrossRefGoogle Scholar
Skellam, J. G. (1949) The probability distribution of gene-differences in relation to selection, mutation, and random extinction. Proc. Camb. Phil. Soc. 45, 364–367.CrossRefGoogle Scholar
Slack, R. S. (1968) A branching process with mean one and possibly infinite variance. Z. Wahrscheinlichkeitsth. 9, 139–145.Google Scholar
Stigum, B. P. (1966) A theorem on the Galton-Watson process. Ann. Math. Statist. 37, 695–698.CrossRefGoogle Scholar
Szekeres, G. (1958) Regular iteration of real and complex functions. Acta Math. 100, 203–258.CrossRefGoogle Scholar
Szekeres, G. (1960) On a theorem of Paul Lévy. Magyar Tud. Akad. Mat. Kutato Int. Közl. A 5, 277–282.Google Scholar
Vere-Jones, D. (1966) Simple stochastic models for the release of quanta of transmitter from a nerve terminal. Aust. J. Statist. 8, 53–63.CrossRefGoogle Scholar
Yaglom, A. M. (1947) Certain limit theorems in the theory of branching stochastic processes. (In Russian.) Doklady Akad. Nauk S.S.S.R. n.s., 56, 795–798.Google Scholar
Zolotarev, V. M. (1957) A refinement of several theorems in the theory of branching stochastic processes. (in Russian.) Teor. Veroyat. Primen. 2, 256–266.Google Scholar
Karamata, J. (1953) Über das asymptotische Verhalten der Folgen die durch Iteration definiert sind. (In Serbian; German summary.) Recueil des Travaux de l'Académie Serbe des Sciences XXXV, Institut Mathématique No. 3, 45–60.Google Scholar
Quine, M. P. and Seneta, E. (1969) A limit theorem tor the Galton-Watson process with immigration. Aust. J. Statist. 11, (to appear).CrossRefGoogle Scholar
Seneta, E. (1968) On recent theorems concerning the supercritical Galton-Watson process. Ann. Math. Statist. 39, 2098–2102.CrossRefGoogle Scholar