Hostname: page-component-6b989bf9dc-g5k2d Total loading time: 0 Render date: 2024-04-14T17:00:07.878Z Has data issue: false hasContentIssue false
  • English
  • Français

SUMS OF POLYNOMIAL-TYPE EXCEPTIONAL UNITS MODULO 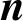$\boldsymbol {n}$

Part of: Sequences and sets Exponential sums and character sums

Published online by Cambridge University Press:  21 July 2021

JUNYONG ZHAO Open the ORCID record for JUNYONG ZHAO [Opens in a new window] ,
SHAOFANG HONG  and
CHAOXI ZHU
JUNYONG ZHAO
Affiliation:
Mathematical College, Sichuan University, Chengdu610064, PR China and School of Mathematics and Statistics, Nanyang Institute of Technology, Nanyang473004, PR China e-mail: zhjy626@163.com
SHAOFANG HONG*
Affiliation:
Mathematical College, Sichuan University, Chengdu610064, PR China e-mail: s-f.hong@tom.com, hongsf02@yahoo.com
CHAOXI ZHU
Affiliation:
Mathematical College, Sichuan University, Chengdu610064, PR China and Science and Technology on Communication Security Laboratory, Chengdu610041, PR China e-mail: zhuxi0824@126.com
*
e-mail: sfhong@scu.edu.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

Let $f(x)\in \mathbb {Z}[x]$ be a nonconstant polynomial. Let 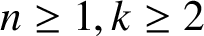$n\ge 1, k\ge 2$ and c be integers. An integer a is called an f-exunit in the ring $\mathbb {Z}_n$ of residue classes modulo n if $\gcd (f(a),n)=1$ . We use the principle of cross-classification to derive an explicit formula for the number 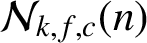${\mathcal N}_{k,f,c}(n)$ of solutions $(x_1,\ldots ,x_k)$ of the congruence 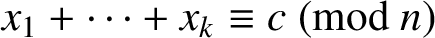$x_1+\cdots +x_k\equiv c\pmod n$ with all 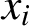$x_i$ being f-exunits in the ring $\mathbb {Z}_n$ . This extends a recent result of Anand et al. 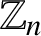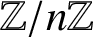[‘On a question of f-exunits in $\mathbb {Z}/{n\mathbb {Z}}$ ’, Arch. Math. (Basel)116 (2021), 403–409]. We derive a more explicit formula for 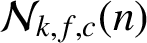${\mathcal N}_{k,f,c}(n)$ when 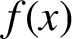$f(x)$ is linear or quadratic.

Keywords

polynomial-type exceptional unitexponential sumring of residue classesprinciple of cross-classification

MSC classification

Primary: 11B13: Additive bases, including sumsets
Secondary: 11L03: Trigonometric and exponential sums, general 11L05: Gauss and Kloosterman sums; generalizations
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 105 , Issue 2 , April 2022 , pp. 202 - 211
Check for updates
Copyright
© 2021 Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

S. F. Hong was partially supported by the National Science Foundation of China, Grant No. 11771304.

References

Anand, J. Chattopadhyay and Roy, B., ‘On sums of polynomial-type exceptional units in $\mathbb{Z}/ \mathrm{n}\mathbb{Z}$ ’, Arch. Math. (Basel) 114 (2020), 271–283.10.1007/s00013-019-01413-7CrossRefGoogle Scholar
Anand, J. Chattopadhyay and Roy, B., ‘On a question of f-exunits in $\mathbb{Z}/ \mathrm{n}\mathbb{Z}$ ’, Arch. Math. (Basel) 116 (2021), 403–409.10.1007/s00013-020-01558-wCrossRefGoogle Scholar
Apostol, T. M., Introduction to Analytic Number Theory (Springer, New York, 1976).Google Scholar
Brauer, A., ‘Lösung der Aufgabe 30’, Jahresber. Dtsch. Math.-Ver. 35 (1926), 92–94.Google Scholar
Lenstra, H. W., ‘Euclidean number fields of large degree’, Invent. Math. 38 (1976--1977), 237–254.10.1007/BF01403131CrossRefGoogle Scholar
Louboutin, S. R., ‘Non-Galois cubic number fields with exceptional units’, Publ. Math. Debrecen 91 (2017), 153–170.10.5486/PMD.2017.7726CrossRefGoogle Scholar
Nagell, T., ‘Sur un type particulier d’unités algébriques, Ark. Mat. 8 (1969), 163–184.10.1007/BF02589556CrossRefGoogle Scholar
Rademacher, H., ‘Aufgabe 30’, Jahresber. Dtsch. Math.-Ver. 34 (1925), 158.Google Scholar
Sander, J. W., ‘On the addition of units and nonunits mod $m$ ’, J. Number Theory 129 (2009), 2260–2266.10.1016/j.jnt.2009.04.010CrossRefGoogle Scholar
Sander, J. W., ‘Sums of exceptional units in residue class rings’, J. Number Theory 159 (2016), 1–6.10.1016/j.jnt.2015.07.018CrossRefGoogle Scholar
Silverman, J. H., ‘Exceptional units and numbers of small Mahler measure’, Exp. Math. 4 (1995), 69–83.10.1080/10586458.1995.10504309CrossRefGoogle Scholar
Silverman, J. H., ‘Small Salem numbers, exceptional units, and Lehmer’s conjecture’, Rocky Mountain J. Math. 26 (1996), 1099–1114.10.1216/rmjm/1181072040CrossRefGoogle Scholar
Smart, N. P., ‘Solving discriminant form equations via unit equations’, J. Symbolic Comput. 21 (1996), 367–374.10.1006/jsco.1996.0018CrossRefGoogle Scholar
Stewart, C. L., ‘Exceptional units and cyclic resultants’, Acta Arith. 155 (2012), 407–418.10.4064/aa155-4-5CrossRefGoogle Scholar
Stewart, C. L., ‘Exceptional units and cyclic resultants, II’, in: Diophantine Methods, Lattices and Arithmetic Theory of Quadratic Forms, Contemporary Mathematics, 587 (American Mathematical Society, Providence, RI, 2013), 191–200.10.1090/conm/587/11698CrossRefGoogle Scholar
Tzanakis, N. and de Weger, B. M. M., ‘On the practical solution of the Thue equation’, J. Number Theory 31 (1989), 99–132.10.1016/0022-314X(89)90014-0CrossRefGoogle Scholar
Tzanakis, N. and de Weger, B. M. M., ‘How to explicitly solve a Thue–Mahler equation’, Compos. Math. 84 (1992), 223–288.Google Scholar
Yang, Q. H. and Zhao, Q. Q., ‘On the sumsets of exceptional units in ${\mathbb{Z}}_n$ ’, Monatsh. Math. 182 (2017), 489–493.10.1007/s00605-015-0872-yCrossRefGoogle Scholar