Hostname: page-component-848d4c4894-4hhp2 Total loading time: 0 Render date: 2024-04-30T17:01:42.048Z Has data issue: false hasContentIssue false
  • English
  • Français

UNIFORM APPROXIMATION BY POLYNOMIAL, RATIONAL AND ANALYTIC FUNCTIONS

Part of: Commutative Banach algebras and commutative topological algebras

Published online by Cambridge University Press:  01 June 2008

T. G. HONARY  and
S. MORADI
T. G. HONARY*
Affiliation:
Faculty of Mathematical Sciences and Computer Engineering, Tarbiat Moallem University, 599 Taleghani Avenue, 15618, Tehran (email: honary@saba.tmu.ac.ir)
S. MORADI
Affiliation:
Faculty of Mathematical Sciences and Computer Engineering, Tarbiat Moallem University, 599 Taleghani Avenue, 15618, Tehran (email: S_moradi@tmu.ac.ir)
*
✉For correspondence; e-mail: honary@saba.tmu.ac.ir
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let K and X be compact plane sets such that . Let P(K) be the uniform closure of polynomials on K, let R(K) be the uniform closure of rational functions on K with no poles in K and let A(K) be the space of continuous functions on K which are analytic on int(K). Define P(X,K),R(X,K) and A(X,K) to be the set of functions in C(X) whose restriction to K belongs to P(K),R(K) and A(K), respectively. Let S0(A) denote the set of peak points for the Banach function algebra A on X. Let S and T be compact subsets of X. We extend the Hartogs–Rosenthal theorem by showing that if the symmetric difference SΔT has planar measure zero, then R(X,S)=R(X,T) . Then we show that the following properties are equivalent:

  1. (i) R(X,S)=R(X,T) ;

  2. (ii) and ;

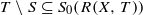  3. (iii) R(K)=C(K) for every compact set ;

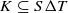  4. (iv) 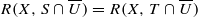for every open set U in ℂ ;

  5. (v) for every p∈X there exists an open disk Dp with centre p such that

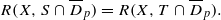
We prove an extension of Vitushkin’s theorem by showing that the following properties are equivalent:

  1. (i) A(X,S)=R(X,T) ;

  2. (ii) for every closed disk 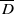in ℂ ;

  3. (iii) for every p∈X there exists an open disk Dp with centre p such that

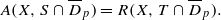
Keywords

uniform algebraspolynomial and rational approximationpeak pointsplanar measureVitushkin’s theorem

MSC classification

Secondary: 46J10: Banach algebras of continuous functions, function algebras 46J15: Banach algebras of differentiable or analytic functions, $H^p$-spaces
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 77 , Issue 3 , June 2008 , pp. 387 - 399
Copyright
Copyright © 2008 Australian Mathematical Society

References

[1]Boivin, A. and Jiang, B., ‘Uniform approximation by meromorphic functions on Riemann surfaces’, J. Anal. Math. 93 (2004), 199–214.CrossRefGoogle Scholar
[2]Gamelin, T. W., Uniform Algebras (Chelsea Publishing Company, New York, 1984).Google Scholar
[3]Gauthier, P. M., ‘Meromorphic uniform approximation on closed subsets of open Riemann surfaces’, in: Approximation Theory and Function Analysis, Proc. Conf. Campinas, 1977 (ed. J. B. Prolla) (North-Holland, Amsterdam, 1979), pp. 139–158.Google Scholar
[4]Hartogs, F. and Rosenthal, A., ‘Über Folgen analytischer Funktionen’, Math. Ann. 104 (1931), 606–610.CrossRefGoogle Scholar
[5]Honary, T. G. and Mahyar, H., ‘Approximation in Lipschitz algebras’, Quaest. Math. 23(1) (2000), 13–19.CrossRefGoogle Scholar
[6]Leibowitz, G. M., Lectures on Complex Function Algebras (Scott, Foresman, Glenview, IL, 1970).Google Scholar
[7]Vitushkin, A. G., ‘Analytic capacity of sets and problems in approximation theory’, Uspekhi Mat. Nauk. 22 (1967), 141–199 (Engl. Transl. Russian Math. Surveys 22 (1967), 139–200).Google Scholar