Hostname: page-component-848d4c4894-nr4z6 Total loading time: 0 Render date: 2024-04-30T21:40:18.525Z Has data issue: false hasContentIssue false
  • English
  • Français

Abel universal functions

Part of: Approximations and expansions Convergence and divergence of infinite limiting processes Universal holomorphic functions General theory of linear operators

Published online by Cambridge University Press:  26 October 2022

Stéphane Charpentier  and
Augustin Mouze
Stéphane Charpentier
Affiliation:
Institut de Mathématiques, UMR 7373, Aix-Marseille Universite, 39 rue F. Joliot Curie, 13453 Cedex 13, Marseille, France e-mail: stephane.charpentier.1@univ-amu.fr
Augustin Mouze*
Affiliation:
Univ.Lille, Centrale Lille - Laboratoire Paul Painlevé, UMR 8524, F-59000 Lille, France
*
e-mail: augustin.mouze@univ-lille.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

Given a sequence 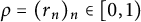$\varrho =(r_n)_n\in [0,1)$ tending to 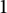$1$, we consider the set 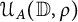${\mathcal {U}}_A({\mathbb {D}},\varrho )$ of Abel universal functions consisting of holomorphic functions f in the open unit disk 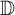$\mathbb {D}$ such that for any compact set K included in the unit circle ${\mathbb {T}}$, different from 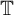${\mathbb {T}}$, the set $\{z\mapsto f(r_n \cdot )\vert _K:n\in \mathbb {N}\}$ is dense in the space 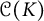${\mathcal {C}}(K)$ of continuous functions on K. It is known that the set ${\mathcal {U}}_A({\mathbb {D}},\varrho )$ is residual in 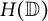$H(\mathbb {D})$. We prove that it does not coincide with any other classical sets of universal holomorphic functions. In particular, it is not even comparable in terms of inclusion to the set of holomorphic functions whose Taylor polynomials at 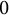$0$ are dense in 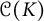${\mathcal {C}}(K)$ for any compact set 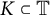$K\subset {\mathbb {T}}$ different from 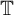${\mathbb {T}}$. Moreover, we prove that the class of Abel universal functions is not invariant under the action of the differentiation operator. Finally, an Abel universal function can be viewed as a universal vector of the sequence of dilation operators 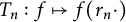$T_n:f\mapsto f(r_n \cdot )$ acting on 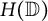$H(\mathbb {D})$. Thus, we study the dynamical properties of 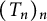$(T_n)_n$ such as the multiuniversality and the (common) frequent universality. All the proofs are constructive.

Keywords

Universal functionsboundary behavioruniversal sequence of operators

MSC classification

Primary: 30K05: Universal Taylor series
Secondary: 40A05: Convergence and divergence of series and sequences 41A10: Approximation by polynomials 47A16: Cyclic vectors, hypercyclic and chaotic operators
Type
Article
Information
Canadian Journal of Mathematics , Volume 75 , Issue 6 , December 2023 , pp. 1957 - 1985
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of The Canadian Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The authors are supported by the grant ANR-17-CE40-0021 of the French National Research Agency ANR (project Front).

References

Abakumov, E., Nestoridis, V., and Picardello, M., Frequently dense harmonic functions and universal martingales on trees . Proc. Amer. Math. Soc. 149(2021), no. 5, 1905–1918.10.1090/proc/15355CrossRefGoogle Scholar
Bayart, F., Universal radial limits of holomorphic functions . Glasg. Math. J. 47(2005), no. 2, 261–267.CrossRefGoogle Scholar
Bayart, F., Boundary behavior and Cesàro means of universal Taylor series . Rev. Mat. Complut. 19(2006), no. 1, 235–247.10.5209/rev_REMA.2006.v19.n1.16662CrossRefGoogle Scholar
Bayart, F., Common hypercyclic vectors for high-dimensional families of operators . Int. Math. Res. Not. IMRN 21(2016), no. 1, 6512–6552.10.1093/imrn/rnv354CrossRefGoogle Scholar
Bayart, F. and Grivaux, S., Frequently hypercyclic operators . Trans. Amer. Math. Soc. 358(2006), 5083–5117.10.1090/S0002-9947-06-04019-0CrossRefGoogle Scholar
Bayart, F., Grosse-Erdmann, K.-G., Nestoridis, V., and Papadimitropoulos, C., Abstract theory of universal series and applications . Proc. Lond. Math. Soc. 96(2008), 417–463.CrossRefGoogle Scholar
Bayart, F. and Matheron, É., Dynamics of linear operators, Cambridge Tracts in Mathematics, 179, Cambridge University Press, Cambridge, 2009.CrossRefGoogle Scholar
Bernal-González, L., Calderón, M. C., and Prado-Bassas, J. A., Maximal cluster sets along arbitrary curves . J. Approx. Theory 129(2004), 207–216.CrossRefGoogle Scholar
Bonilla, A. and Grosse-Erdmann, K.-G., Frequently hypercyclic operators and vectors . Ergodic Theory Dynam. Systems 27(2007), no. 2, 383–404.10.1017/S014338570600085XCrossRefGoogle Scholar
Bourdon, P., Somewhere dense orbits are everywhere dense . Indiana Univ. Math. J. 52(2003), no. 3, 811–819.10.1512/iumj.2003.52.2303CrossRefGoogle Scholar
Charpentier, S., On countably universal series in the complex plane . Complex Var. Elliptic Equ. 64(2019), no. 6, 1025–1042.CrossRefGoogle Scholar
Charpentier, S., Holomorphic functions with universal boundary behaviour . J. Approx. Theory 254(2020), 105391.CrossRefGoogle Scholar
Charpentier, S., Ernst, R., Mestiri, M., and Mouze, A., Common frequent hypercyclicity . J. Funct. Anal. 283(2022), no. 3, Article no. 109526, 51 pp.CrossRefGoogle Scholar
Charpentier, S. and Kosiński, L., Wild boundary behaviour of holomorphic functions in domains of  ${\mathbb{C}}^N$ . Indiana Univ. Math. J. 70(2021), no. 6, 2351–2367.10.1512/iumj.2021.70.8749CrossRefGoogle Scholar
Charpentier, S. and Mouze, A., Universal Taylor series and summability . Rev. Mat. Complut. 28(2015), no. 1, 153–167.CrossRefGoogle Scholar
Charpentier, S. and Mouze, A., Universal sequences of composition operators. Preprint, 2021. arXiv:2111.05165v1 Google Scholar
Chui, C. K. and Parnes, M. N., Approximation by overconvergence of power series . J. Math. Anal. Appl. 36(1971), 693–696.CrossRefGoogle Scholar
Conejero, J. A., Peris, A., and Müller, V., Hypercyclic behaviour of operators in a hypercyclic ${C}_0$ -semigroup. J. Funct. Anal. 244(2007), no. 1, 342–348.10.1016/j.jfa.2006.12.008CrossRefGoogle Scholar
Costakis, G., Some remarks on universal functions and Taylor series . Math. Proc. Camb. Philos. Soc. 128(2000), no. 1, 157–175.10.1017/S0305004199003886CrossRefGoogle Scholar
Costakis, G., On the radial behavior of universal Taylor series . Monatsh. Math. 145(2005), 11–17.CrossRefGoogle Scholar
Costakis, G., On a conjecture of D. Herrero concerning hypercyclic operators . C. R. Acad. Sci. Paris Sér. I Math. 330(2000), no. 3, 179–182.CrossRefGoogle Scholar
Costakis, G., Jung, A., and Müller, J., Generic behavior of classes of Taylor series outside the unit disk . Constr. Approx. 49(2019), no. 3, 509–524.CrossRefGoogle Scholar
Costakis, G. and Tsirivas, N., Doubly universal Taylor series . J. Approx. Theory 180(2014), 21–31.10.1016/j.jat.2013.12.006CrossRefGoogle Scholar
Gardiner, S. J., Boundary behaviour of functions which possess universal Taylor series . Bull. Lond. Math. Soc. 45(2013), no. 1, 191–199.10.1112/blms/bds078CrossRefGoogle Scholar
Gardiner, S. J. and Manolaki, M., Boundary behaviour of universal Taylor series on multiply connected domains . Constr. Approx. 40(2014), no. 2, 259–279.10.1007/s00365-014-9237-3CrossRefGoogle Scholar
Gehlen, W., Luh, W., and Müller, J., On the existence of O-universal functions . Complex Variables Theory Appl. 41(2000), no. 1, 81–90.10.1080/17476930008815238CrossRefGoogle Scholar
Grivaux, S., Matheron, E., and Menet, Q., Linear dynamical systems on Hilbert spaces: typical properties and explicit examples . Mem. Amer. Math. Soc. 269(2021), no. 1315, v + 147 pp.Google Scholar
Grosse Erdmann, K.-G., Universal families and hypercyclic operators . Bull. Amer. Math. Soc. (N.S.) 36(1999), no. 3, 345–381.CrossRefGoogle Scholar
Grosse-Erdmann, K.-G. and Peris Manguillot, A., Linear chaos, Universitext, Springer, London, 2011.10.1007/978-1-4471-2170-1CrossRefGoogle Scholar
Kierst, S. and Szpilrajn, D., Sur certaines singularités des fonctions analytiques uniformes . Fundam. Math. 21(1933), 276–294.CrossRefGoogle Scholar
Luh, W., Approximation analytischer Funktionen durch uberkonvergente Potenzreihen und deren matrix-Transformierten . Mitt. Math. Sem. Giessen. 88(1970), 1–56.Google Scholar
Maronikolakis, K., Universal radial approximation in spaces of analytic functions . J. Math. Anal. Appl. 512(2022), no. 1, 13.10.1016/j.jmaa.2022.126102CrossRefGoogle Scholar
Melas, A. and Nestoridis, V., Universality of Taylor series as a generic property of holomorphic functions . Adv. Math. 157(2001), 138–176.10.1006/aima.2000.1955CrossRefGoogle Scholar
Melas, A., Nestoridis, V., and Papadoperakis, I., Growth of coefficients of universal Taylor series and comparison of two classes of functions . J. Anal. Math. 73(1997), 187–202.CrossRefGoogle Scholar
Mouze, A., Multi-universal series . Arch. Math. 115(2020), no. 1, 79–88.10.1007/s00013-020-01467-yCrossRefGoogle Scholar
Mouze, A., Universal Taylor series with respect to a prescribed subsequence . J. Math. Anal. Appl. 498(2021), no. 1, Article no. 124953, 14 pp.10.1016/j.jmaa.2021.124953CrossRefGoogle Scholar
Mouze, A. and Munnier, V., Polynomial inequalities and universal Taylor series . Math. Z. 284(2016), nos. 3–4, 919–946.10.1007/s00209-016-1679-9CrossRefGoogle Scholar
Mouze, A. and Munnier, V., On the frequent universality of universal Taylor series in the complex plane . Glasg. Math. J. 59(2017), no. 1, 109–117.10.1017/S0017089516000069CrossRefGoogle Scholar
Nestoridis, V., Universal Taylor series . Ann. Inst. Fourier (Grenoble) 46(1996), no. 5, 1293–1306.10.5802/aif.1549CrossRefGoogle Scholar
Peris, A., Multihypercyclic operators are hypercyclic . Math. Z. 236(2001), 779–786.CrossRefGoogle Scholar
Shkarin, S., On the spectrum of frequently hypercyclic operators . Proc. Amer. Math. Soc. 137(2009), no. 1, 123–134.10.1090/S0002-9939-08-09655-XCrossRefGoogle Scholar
Zygmund, A., Trigonometric series, Cambridge University Press, Cambridge, 1979.Google Scholar