Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Banica, Teodor
and
Speicher, Roland
2009.
Liberation of orthogonal Lie groups.
Advances in Mathematics,
Vol. 222,
Issue. 4,
p.
1461.
Jarosz, Andrzej
2011.
Summing free unitary random matrices.
Physical Review E,
Vol. 84,
Issue. 1,
Penson, Karol A.
and
Życzkowski, Karol
2011.
Product of Ginibre matrices: Fuss-Catalan and Raney distributions.
Physical Review E,
Vol. 83,
Issue. 6,
Życzkowski, Karol
Penson, Karol A.
Nechita, Ion
and
Collins, Benoît
2011.
Generating random density matrices.
Journal of Mathematical Physics,
Vol. 52,
Issue. 6,
Banica, T.
and
Skalski, A.
2011.
Quantum Isometry Groups of Duals of Free Powers of Cyclic Groups.
International Mathematics Research Notices,
Burda, Z.
Nowak, M. A.
and
Swiech, A.
2012.
Spectral relations between products and powers of isotropic random matrices.
Physical Review E,
Vol. 86,
Issue. 6,
Pérez-Abreu, Victor
and
Sakuma, Noriyoshi
2012.
Free Infinite Divisibility of Free Multiplicative Mixtures of the Wigner Distribution.
Journal of Theoretical Probability,
Vol. 25,
Issue. 1,
p.
100.
Ortmann, Janosch
2012.
Large deviations for non-crossing partitions.
Electronic Journal of Probability,
Vol. 17,
Issue. none,
Zhang, Lun
2013.
A note on the limiting mean distribution of singular values for products of two Wishart random matrices.
Journal of Mathematical Physics,
Vol. 54,
Issue. 8,
Banica, Teodor
and
Nechita, Ion
2013.
Asymptotic Eigenvalue Distributions of Block-Transposed Wishart Matrices.
Journal of Theoretical Probability,
Vol. 26,
Issue. 3,
p.
855.
Akemann, Gernot
Ipsen, Jesper R.
and
Kieburg, Mario
2013.
Products of rectangular random matrices: Singular values and progressive scattering.
Physical Review E,
Vol. 88,
Issue. 5,
Haagerup, Uffe
and
Möller, Sören
2013.
Operator Algebra and Dynamics.
Vol. 58,
Issue. ,
p.
157.
Akemann, Gernot
Kieburg, Mario
and
Wei, Lu
2013.
Singular value correlation functions for products of Wishart random matrices.
Journal of Physics A: Mathematical and Theoretical,
Vol. 46,
Issue. 27,
p.
275205.
Forrester, Peter J
2014.
Eigenvalue statistics for product complex Wishart matrices.
Journal of Physics A: Mathematical and Theoretical,
Vol. 47,
Issue. 34,
p.
345202.
Lenczewski, Romuald
2014.
Limit distributions of random matrices.
Advances in Mathematics,
Vol. 263,
Issue. ,
p.
253.
Lenczewski, R.
2014.
Random Matrix Model for Free Meixner Laws.
International Mathematics Research Notices,
Kuijlaars, Arno B. J.
and
Zhang, Lun
2014.
Singular Values of Products of Ginibre Random Matrices, Multiple Orthogonal Polynomials and Hard Edge Scaling Limits.
Communications in Mathematical Physics,
Vol. 332,
Issue. 2,
p.
759.
Lemeux, François
2014.
The fusion rules of some free wreath product quantum groups and applications.
Journal of Functional Analysis,
Vol. 267,
Issue. 7,
p.
2507.
Lemeux, François
2015.
Haagerup approximation property for quantum reflection groups.
Proceedings of the American Mathematical Society,
Vol. 143,
Issue. 5,
p.
2017.
Zhang, Lun
2015.
Local Universality in Biorthogonal Laguerre Ensembles.
Journal of Statistical Physics,
Vol. 161,
Issue. 3,
p.
688.
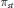 ${{\pi }_{st}}$ that we call free Bessel laws. These are related to the free Poisson law
${{\pi }_{st}}$ that we call free Bessel laws. These are related to the free Poisson law  $\pi $ via the formulae
$\pi $ via the formulae 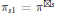 ${{\text{ }\!\!\pi\!\!\text{ }}_{s1}}={{\text{ }\!\!\pi\!\!\text{ }}^{\boxtimes s}}$ and
${{\text{ }\!\!\pi\!\!\text{ }}_{s1}}={{\text{ }\!\!\pi\!\!\text{ }}^{\boxtimes s}}$ and  $\text{ }\pi {{\text{ }}_{1t}}=\text{ }\pi {{\text{ }}^{\boxtimes }}^{t}$ . Our study includes definition and basic properties, analytic aspects (supports, atoms, densities), combinatorial aspects (functional transforms, moments, partitions), and a discussion of the relation with random matrices and quantum groups.
$\text{ }\pi {{\text{ }}_{1t}}=\text{ }\pi {{\text{ }}^{\boxtimes }}^{t}$ . Our study includes definition and basic properties, analytic aspects (supports, atoms, densities), combinatorial aspects (functional transforms, moments, partitions), and a discussion of the relation with random matrices and quantum groups.