No CrossRef data available.
Article contents
Linear independence of series related to the Thue–Morse sequence along powers
Part of:
Sequences and sets
Elementary number theory
Diophantine approximation, transcendental number theory
Published online by Cambridge University Press: 06 March 2024
Abstract
The Thue–Morse sequence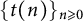 
$\{t(n)\}_{n\geqslant 0}$ is the indicator function of the parity of the number of ones in the binary expansion of nonnegative integers n, where 
$t(n)=1$ (resp.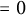 
$=0$) if the binary expansion of n has an odd (resp. even) number of ones. In this paper, we generalize a recent result of E. Miyanohara by showing that, for a fixed Pisot or Salem number 
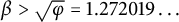$\beta>\sqrt {\varphi }=1.272019\ldots $, the set of the numbers is linearly independent over the field 
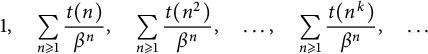$$\begin{align*}1,\quad \sum_{n\geqslant1}\frac{t(n)}{\beta^{n}},\quad \sum_{n\geqslant1}\frac{t(n^2)}{\beta^{n}},\quad \dots, \quad \sum_{n\geqslant1}\frac{t(n^k)}{\beta^{n}},\quad \dots \end{align*}$$
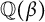$\mathbb {Q}(\beta )$, where 
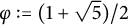$\varphi :=(1+\sqrt {5})/2$ is the golden ratio. Our result yields that for any integer 
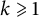$k\geqslant 1$ and for any 
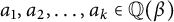$a_1,a_2,\ldots ,a_k\in \mathbb {Q}(\beta )$, not all zero, the sequence {
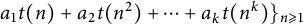$a_1t(n)+a_2t(n^2)+\cdots +a_kt(n^k)\}_{n\geqslant 1}$ cannot be eventually periodic.
MSC classification
- Type
- Article
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press on behalf of Canadian Mathematical Society
Footnotes
This work was supported by the Research Institute for Mathematical Sciences, an International Joint Usage/Research Center located in Kyoto University. This research was partly supported by JSPS KAKENHI Grant Number JP22K03263.
References
Allouche, J.-P. and Shallit, J., Automatic sequences: theory, applications, generalizations, Cambridge University Press, Cambridge, 2003.CrossRefGoogle Scholar
Drmota, M., Mauduit, C., and Rivat, J.,
Normality along squares
. J. Eur. Math. Soc. 21(2019), no. 2, 507–548.CrossRefGoogle Scholar
Mahler, K.,
Arithmetische Eigenschaften der Lösungen einer Klasse von Funktionalgleichungen
. Math. Ann. 101(1929), 342–366. Corrigendum 103(1930), 532.CrossRefGoogle Scholar
Miyanohara, E.,
An arithmetical property of the real numbers generated by Thue–Morse sequence along squares
. Acta Math. Hungar. 170(2023), 430–435.CrossRefGoogle Scholar
Moshe, Y.,
On the subword complexity of Thue–Morse polynomial extractions
. Theor. Comput. Sci. 389(2007), 318–329.CrossRefGoogle Scholar
Prouhet, E.,
Mémoire Sur quelques relations entre les puissances des nombres
. C. R. Acad. Sci. Paris Sér. I 33(1851), 225.Google Scholar
Spiegelhofer, L., Thue–Morse along the sequence of cubes. Preprint, 2023, arXiv:2308.09498 [math.NT].Google Scholar
Thue, A.,
Über unendliche Zeichenreihen
. Norske vid. Selsk. Skr. Mat. Nat. Kl. 7(1906), 1–22. Reprinted in: T. Nagell (Ed.), Selected mathematical papers of Axel Thue, Universitetsforlaget, Oslo, 1977, pp. 139–158.Google Scholar
Thue, A.,
Über die gegenseitige Lage gleicher Teile gewisser Zeichenreihen
. Norske vid. Selsk. Skr. Mat. Nat. Kl. 1(1912), 1–67. Reprinted in: T. Nagell (Ed.), Selected mathematical papers of Axel Thue, Universitetsforlaget, Oslo, 1977, pp. 413–478.Google Scholar