Published online by Cambridge University Press: 07 January 2019
Let 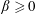 $\unicode[STIX]{x1D6FD}\geqslant 0$, let
$\unicode[STIX]{x1D6FD}\geqslant 0$, let  $e_{1}=(1,0,\ldots ,0)$ be a unit vector on
$e_{1}=(1,0,\ldots ,0)$ be a unit vector on 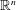 $\mathbb{R}^{n}$, and let
$\mathbb{R}^{n}$, and let 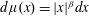 $d\unicode[STIX]{x1D707}(x)=|x|^{\unicode[STIX]{x1D6FD}}dx$ be a power weighted measure on
$d\unicode[STIX]{x1D707}(x)=|x|^{\unicode[STIX]{x1D6FD}}dx$ be a power weighted measure on  $\mathbb{R}^{n}$. For
$\mathbb{R}^{n}$. For 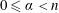 $0\leqslant \unicode[STIX]{x1D6FC}<n$, let
$0\leqslant \unicode[STIX]{x1D6FC}<n$, let 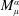 $M_{\unicode[STIX]{x1D707}}^{\unicode[STIX]{x1D6FC}}$ be the centered Hardy-Littlewood maximal function and fractional maximal functions associated with measure
$M_{\unicode[STIX]{x1D707}}^{\unicode[STIX]{x1D6FC}}$ be the centered Hardy-Littlewood maximal function and fractional maximal functions associated with measure 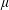 $\unicode[STIX]{x1D707}$. This paper shows that for
$\unicode[STIX]{x1D707}$. This paper shows that for 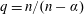 $q=n/(n-\unicode[STIX]{x1D6FC})$,
$q=n/(n-\unicode[STIX]{x1D6FC})$, 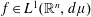 $f\in L^{1}(\mathbb{R}^{n},d\unicode[STIX]{x1D707})$,
$f\in L^{1}(\mathbb{R}^{n},d\unicode[STIX]{x1D707})$, 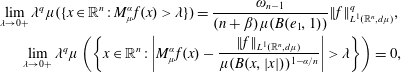 $$\begin{eqnarray}\displaystyle \lim _{\unicode[STIX]{x1D706}\rightarrow 0+}\unicode[STIX]{x1D706}^{q}\unicode[STIX]{x1D707}(\{x\in \mathbb{R}^{n}:M_{\unicode[STIX]{x1D707}}^{\unicode[STIX]{x1D6FC}}f(x)>\unicode[STIX]{x1D706}\})=\frac{\unicode[STIX]{x1D714}_{n-1}}{(n+\unicode[STIX]{x1D6FD})\unicode[STIX]{x1D707}(B(e_{1},1))}\Vert f\Vert _{L^{1}(\mathbb{R}^{n},d\unicode[STIX]{x1D707})}^{q}, & & \displaystyle \nonumber\\ \displaystyle \lim _{\unicode[STIX]{x1D706}\rightarrow 0+}\unicode[STIX]{x1D706}^{q}\unicode[STIX]{x1D707}\left(\left\{x\in \mathbb{R}^{n}:\left|M_{\unicode[STIX]{x1D707}}^{\unicode[STIX]{x1D6FC}}f(x)-\frac{\Vert f\Vert _{L^{1}(\mathbb{R}^{n},d\unicode[STIX]{x1D707})}}{\unicode[STIX]{x1D707}(B(x,|x|))^{1-\unicode[STIX]{x1D6FC}/n}}\right|>\unicode[STIX]{x1D706}\right\}\right)=0, & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \lim _{\unicode[STIX]{x1D706}\rightarrow 0+}\unicode[STIX]{x1D706}^{q}\unicode[STIX]{x1D707}(\{x\in \mathbb{R}^{n}:M_{\unicode[STIX]{x1D707}}^{\unicode[STIX]{x1D6FC}}f(x)>\unicode[STIX]{x1D706}\})=\frac{\unicode[STIX]{x1D714}_{n-1}}{(n+\unicode[STIX]{x1D6FD})\unicode[STIX]{x1D707}(B(e_{1},1))}\Vert f\Vert _{L^{1}(\mathbb{R}^{n},d\unicode[STIX]{x1D707})}^{q}, & & \displaystyle \nonumber\\ \displaystyle \lim _{\unicode[STIX]{x1D706}\rightarrow 0+}\unicode[STIX]{x1D706}^{q}\unicode[STIX]{x1D707}\left(\left\{x\in \mathbb{R}^{n}:\left|M_{\unicode[STIX]{x1D707}}^{\unicode[STIX]{x1D6FC}}f(x)-\frac{\Vert f\Vert _{L^{1}(\mathbb{R}^{n},d\unicode[STIX]{x1D707})}}{\unicode[STIX]{x1D707}(B(x,|x|))^{1-\unicode[STIX]{x1D6FC}/n}}\right|>\unicode[STIX]{x1D706}\right\}\right)=0, & & \displaystyle \nonumber\end{eqnarray}$$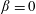 $\unicode[STIX]{x1D6FD}=0$. Meanwhile, the corresponding results for the un-centered maximal functions as well as the fractional integral operators with respect to measure
$\unicode[STIX]{x1D6FD}=0$. Meanwhile, the corresponding results for the un-centered maximal functions as well as the fractional integral operators with respect to measure 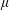 $\unicode[STIX]{x1D707}$ are also obtained.
$\unicode[STIX]{x1D707}$ are also obtained.
Supported by the NNSF of China (Nos. 11771358, 11471041) and the NSF of Fujian Province of China (No. 2015J01025). Huoxiong Wu is the corresponding author.