Let R be an integral domain with $qf(R)=K$ , and let 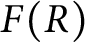$F(R)$ be the set of nonzero fractional ideals of R. Call R a dually compact domain (DCD) if, for each 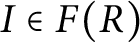$I\in F(R)$ , the ideal 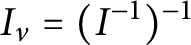$I_{v}=(I^{-1})^{-1}$ is a finite intersection of principal fractional ideals. We characterize DCDs and show that the class of DCDs properly contains various classes of integral domains, such as Noetherian, Mori, and Krull domains. In addition, we show that a Schreier DCD is a greatest common divisor (GCD) (Greatest Common Divisor) domain with the property that, for each 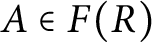$A\in F(R)$ , the ideal $A_{v}$ is principal. We show that a domain R is G-Dedekind (i.e., has the property that 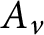$A_{v}$ is invertible for each 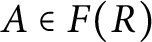$A\in F(R)$ ) if and only if R is a DCD satisfying the property $\ast :$ For all pairs of subsets 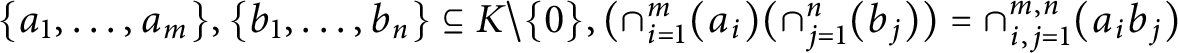$\{a_{1},\ldots ,a_{m}\},\{b_{1},\ldots ,b_{n}\}\subseteq K\backslash \{0\}, (\cap _{i=1}^{m}(a_{i})(\cap _{j=1}^{n}(b_{j}))=\cap _{i,j=1}^{m,n}(a_{i}b_{j})$ . We discuss what the appropriate names for G-Dedekind domains and related notions should be. We also make some observations about how the DCDs behave under localizations and polynomial ring extensions.