Article contents
Uniform s-Cross-Intersecting Families
Published online by Cambridge University Press: 28 March 2017
Abstract
In this paper we study a question related to the classical Erdős–Ko–Rado theorem, which states that any family of k-element subsets of the set [n] = {1,. . .,n} in which any two sets intersect has cardinality at most 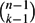 $\binom{n-1}{k-1}$ .
$\binom{n-1}{k-1}$ .
We say that two non-empty families  ${\mathcal A}, {\mathcal B}\subset \binom{[n]}{k}$ are s-cross-intersecting if, for any A ∈
${\mathcal A}, {\mathcal B}\subset \binom{[n]}{k}$ are s-cross-intersecting if, for any A ∈ 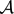 ${\mathcal A}$ , B ∈
${\mathcal A}$ , B ∈  ${\mathcal B}$ , we have |A ∩ B| ≥ s. In this paper we determine the maximum of |
${\mathcal B}$ , we have |A ∩ B| ≥ s. In this paper we determine the maximum of | 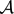 ${\mathcal A}$ |+|
${\mathcal A}$ |+|  ${\mathcal B}$ | for all n. This generalizes a result of Hilton and Milner, who determined the maximum of |
${\mathcal B}$ | for all n. This generalizes a result of Hilton and Milner, who determined the maximum of | 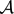 ${\mathcal A}$ |+|
${\mathcal A}$ |+|  ${\mathcal B}$ | for non-empty 1-cross-intersecting families.
${\mathcal B}$ | for non-empty 1-cross-intersecting families.
Keywords
Information
- Type
- Paper
- Information
- Copyright
- Copyright © Cambridge University Press 2017
References
- 9
- Cited by

