Published online by Cambridge University Press: 26 March 2020
For  ${\mathcal{C}}$ a factorisable and pivotal finite tensor category over an algebraically closed field of characteristic zero we show:
${\mathcal{C}}$ a factorisable and pivotal finite tensor category over an algebraically closed field of characteristic zero we show:
(1) 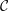 ${\mathcal{C}}$ always contains a simple projective object;
${\mathcal{C}}$ always contains a simple projective object;
(2) if 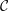 ${\mathcal{C}}$ is in addition ribbon, the internal characters of projective modules span a submodule for the projective
${\mathcal{C}}$ is in addition ribbon, the internal characters of projective modules span a submodule for the projective  $\text{SL}(2,\mathbb{Z})$-action;
$\text{SL}(2,\mathbb{Z})$-action;
(3) the action of the Grothendieck ring of  ${\mathcal{C}}$ on the span of internal characters of projective objects can be diagonalised;
${\mathcal{C}}$ on the span of internal characters of projective objects can be diagonalised;
(4) the linearised Grothendieck ring of 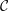 ${\mathcal{C}}$ is semisimple if and only if
${\mathcal{C}}$ is semisimple if and only if 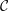 ${\mathcal{C}}$ is semisimple.
${\mathcal{C}}$ is semisimple.
Results (1)–(3) remain true in positive characteristic under an extra assumption. Result (1) implies that the tensor ideal of projective objects in 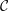 ${\mathcal{C}}$ carries a unique-up-to-scalars modified trace function. We express the modified trace of open Hopf links coloured by projectives in terms of
${\mathcal{C}}$ carries a unique-up-to-scalars modified trace function. We express the modified trace of open Hopf links coloured by projectives in terms of 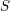 $S$-matrix elements. Furthermore, we give a Verlinde-like formula for the decomposition of tensor products of projective objects which uses only the modular
$S$-matrix elements. Furthermore, we give a Verlinde-like formula for the decomposition of tensor products of projective objects which uses only the modular 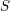 $S$-transformation restricted to internal characters of projective objects. We compute the modified trace in the example of symplectic fermion categories, and we illustrate how the Verlinde-like formula for projective objects can be applied there.
$S$-transformation restricted to internal characters of projective objects. We compute the modified trace in the example of symplectic fermion categories, and we illustrate how the Verlinde-like formula for projective objects can be applied there.