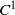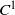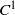Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Bessa, Mário
2013.
On $$C^1$$ -Generic Chaotic Systems in Three-Manifolds.
Qualitative Theory of Dynamical Systems,
Vol. 12,
Issue. 2,
p.
323.
Lee, Keonhee
and
Lee, Manseob
2013.
Divergence-free vector fields with inverse shadowing.
Advances in Difference Equations,
Vol. 2013,
Issue. 1,
Bessa, Mário
Lee, Manseob
and
Vaz, Sandra
2014.
Stable weakly shadowable volume-preserving systems are volume-hyperbolic.
Acta Mathematica Sinica, English Series,
Vol. 30,
Issue. 6,
p.
1007.
Ribeiro, Raquel
2014.
Hyperbolicity and types of shadowing for $C^1$ generic vector fields.
Discrete & Continuous Dynamical Systems - A,
Vol. 34,
Issue. 7,
p.
2963.
Bessa, Mário
Carvalho, Maria
and
Rodrigues, Alexandre
2015.
Generic area-preserving reversible diffeomorphisms.
Nonlinearity,
Vol. 28,
Issue. 6,
p.
1695.
Yang, Jiagang
and
Zhou, Yunhua
2015.
Generic continuity of metric entropy for volume-preserving diffeomorphisms.
Journal of Mathematical Analysis and Applications,
Vol. 425,
Issue. 1,
p.
364.
Morales, C.A.
2016.
A characterization of singular-hyperbolicity.
Michigan Mathematical Journal,
Vol. 65,
Issue. 2,
Barragán, Andrés Mauricio
and
Morales, Carlos Arnoldo
2016.
On ergodic measures with negative Lyapunov exponents.
Proceedings of the Japan Academy, Series A, Mathematical Sciences,
Vol. 92,
Issue. 10,
Bessa, Mário
and
Carvalho, Glória Ferreira
2019.
The Lyapunov exponents of generic skew-product compact semiflows.
Journal of Evolution Equations,
Vol. 19,
Issue. 2,
p.
387.
Wu, Xinxing
Zhang, Xu
and
Sun, Fu
2021.
Volume-Preserving Diffeomorphisms with the $$\mathcal {M}_0$$-Shadowing Properties.
Mediterranean Journal of Mathematics,
Vol. 18,
Issue. 2,
Bessa, Mário
2023.
Lyapunov exponents and entropy for divergence-free Lipschitz vector fields.
European Journal of Mathematics,
Vol. 9,
Issue. 2,