Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Li, Ping
2011.
The rigidity of Dolbeault-type operators and symplectic circle actions.
Proceedings of the American Mathematical Society,
Vol. 140,
Issue. 6,
p.
1987.
Li, Ping
2012.
Circle action, lower bound of fixed points and characteristic numbers.
Journal of Fixed Point Theory and Applications,
Vol. 11,
Issue. 2,
p.
245.
Кустарев, Андрей Александрович
and
Kustarev, Andrei Aleksandrovich
2013.
Почти комплексные действия окружности с малым числом неподвижных точек.
Успехи математических наук,
Vol. 68,
Issue. 3(411),
p.
191.
Kustarev, A A
2013.
Almost complex circle actions with few fixed points.
Russian Mathematical Surveys,
Vol. 68,
Issue. 3,
p.
574.
JANG, DONGHOON
2014.
Symplectic periodic flows with exactly three equilibrium points.
Ergodic Theory and Dynamical Systems,
Vol. 34,
Issue. 6,
p.
1930.
Godinho, Leonor
and
Sabatini, Silvia
2014.
New Tools for Classifying Hamiltonian Circle Actions with Isolated Fixed Points.
Foundations of Computational Mathematics,
Vol. 14,
Issue. 4,
p.
791.
Li, Ping
2016.
Eigenvalues of vector fields, Bott's residue formula and integral invariants.
Differential Geometry and its Applications,
Vol. 44,
Issue. ,
p.
122.
Jang, Donghoon
2017.
Circle actions on almost complex manifolds with isolated fixed points.
Journal of Geometry and Physics,
Vol. 119,
Issue. ,
p.
187.
Tolman, Susan
2017.
Non-Hamiltonian actions with isolated fixed points.
Inventiones mathematicae,
Vol. 210,
Issue. 3,
p.
877.
Jang, Donghoon
2018.
Circle actions on oriented manifolds with discrete fixed point sets and classification in dimension 4.
Journal of Geometry and Physics,
Vol. 133,
Issue. ,
p.
181.
Bolsinov, Alexey
Matveev, Vladimir S.
Miranda, Eva
and
Tabachnikov, Serge
2018.
Open problems, questions and challenges in finite- dimensional integrable systems.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 376,
Issue. 2131,
p.
20170430.
Wen, Shiyun
and
Ma, Jun
2019.
Number of fixed points for unitary Tn−1-manifold.
Frontiers of Mathematics in China,
Vol. 14,
Issue. 4,
p.
819.
Jang, Donghoon
2020.
Circle actions on 8-dimensional almost complex manifolds with 4 fixed points.
Journal of Fixed Point Theory and Applications,
Vol. 22,
Issue. 4,
Jang, Donghoon
2020.
Circle actions on almost complex manifolds with 4 fixed points.
Mathematische Zeitschrift,
Vol. 294,
Issue. 1-2,
p.
287.
Jang, Donghoon
and
Tolman, Susan
2023.
Non-Hamiltonian Actions With Fewer Isolated Fixed Points.
International Mathematics Research Notices,
Vol. 2023,
Issue. 7,
p.
6045.
Jang, Donghoon
2023.
Circle actions on 6-dimensional oriented manifolds with 4 fixed points.
Journal of Fixed Point Theory and Applications,
Vol. 25,
Issue. 3,
Jang, Donghoon
2024.
Circle Actions on Oriented Manifolds With 3 Fixed Points.
International Mathematics Research Notices,
Vol. 2024,
Issue. 15,
p.
11371.
Wiemeler, Michael
2025.
On circle actions with exactly three fixed points.
Journal of Fixed Point Theory and Applications,
Vol. 27,
Issue. 3,
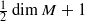 fixed points; this follows from Morse theory for the momentum map of the action. In this paper we use Atiyah–Bott–Berline–Vergne (ABBV) localization in equivariant cohomology to prove that this conclusion also holds for symplectic circle actions with non-empty fixed sets, as long as the Chern class map is somewhere injective—the Chern class map assigns to a fixed point the sum of the action weights at the point. We complement this result with less sharp lower bounds on the number of fixed points, under no assumptions; from a dynamical systems viewpoint, our results imply that there is no symplectic periodic flow with exactly one or two equilibrium points on a compact manifold of dimension at least eight.
fixed points; this follows from Morse theory for the momentum map of the action. In this paper we use Atiyah–Bott–Berline–Vergne (ABBV) localization in equivariant cohomology to prove that this conclusion also holds for symplectic circle actions with non-empty fixed sets, as long as the Chern class map is somewhere injective—the Chern class map assigns to a fixed point the sum of the action weights at the point. We complement this result with less sharp lower bounds on the number of fixed points, under no assumptions; from a dynamical systems viewpoint, our results imply that there is no symplectic periodic flow with exactly one or two equilibrium points on a compact manifold of dimension at least eight.
