Article contents
Foliated hyperbolicity and foliations with hyperbolic leaves
Published online by Cambridge University Press: 17 September 2018
Abstract
Given a lamination in a compact space and a laminated vector field 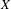 $X$ which is hyperbolic when restricted to the leaves of the lamination, we distinguish a class of
$X$ which is hyperbolic when restricted to the leaves of the lamination, we distinguish a class of 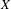 $X$-invariant probabilities that describe the behavior of almost every
$X$-invariant probabilities that describe the behavior of almost every 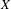 $X$-orbit in every leaf, which we call Gibbs
$X$-orbit in every leaf, which we call Gibbs  $u$-states. We apply this to the case of foliations in compact manifolds having leaves with negative curvature, using the foliated hyperbolic vector field on the unit tangent bundle to the foliation generating the leaf geodesics. When the Lyapunov exponents of such ergodic Gibbs
$u$-states. We apply this to the case of foliations in compact manifolds having leaves with negative curvature, using the foliated hyperbolic vector field on the unit tangent bundle to the foliation generating the leaf geodesics. When the Lyapunov exponents of such ergodic Gibbs  $u$-states are negative, it is an SRB measure (having a positive Lebesgue basin of attraction). When the foliation is by hyperbolic leaves, this class of probabilities coincide with the classical harmonic measures introduced by Garnett. Furthermore, if the foliation is transversally conformal and does not admit a transverse invariant measure we show that there are finitely many ergodic Gibbs
$u$-states are negative, it is an SRB measure (having a positive Lebesgue basin of attraction). When the foliation is by hyperbolic leaves, this class of probabilities coincide with the classical harmonic measures introduced by Garnett. Furthermore, if the foliation is transversally conformal and does not admit a transverse invariant measure we show that there are finitely many ergodic Gibbs  $u$-states, each supported in one minimal set of the foliation, each having negative Lyapunov exponents, and the union of their basins of attraction has full Lebesgue measure. The leaf geodesics emanating from a point have a proportion whose asymptotic statistics are described by each of these ergodic Gibbs
$u$-states, each supported in one minimal set of the foliation, each having negative Lyapunov exponents, and the union of their basins of attraction has full Lebesgue measure. The leaf geodesics emanating from a point have a proportion whose asymptotic statistics are described by each of these ergodic Gibbs  $u$-states, giving rise to continuous visibility functions of the attractors. Reversing time, by considering
$u$-states, giving rise to continuous visibility functions of the attractors. Reversing time, by considering 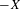 $-X$, we obtain the existence of the same number of repellers of the foliated geodesic flow having the same harmonic measures as projections to
$-X$, we obtain the existence of the same number of repellers of the foliated geodesic flow having the same harmonic measures as projections to 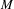 $M$. In the case of only one attractor, we obtain a north to south pole dynamics.
$M$. In the case of only one attractor, we obtain a north to south pole dynamics.
Information
- Type
- Original Article
- Information
- Copyright
- © Cambridge University Press, 2018
References
- 4
- Cited by

