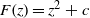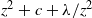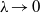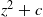Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
XIAO, YINGQING
QIU, WEIYUAN
and
YIN, YONGCHENG
2014.
On the dynamics of generalized McMullen maps.
Ergodic Theory and Dynamical Systems,
Vol. 34,
Issue. 6,
p.
2093.
Devaney, Robert L.
2016.
Exotic topology in complex dynamics.
Indagationes Mathematicae,
Vol. 27,
Issue. 5,
p.
1116.
Werbos, Paul J.
2016.
Cognitive Phase Transitions in the Cerebral Cortex - Enhancing the Neuron Doctrine by Modeling Neural Fields.
Vol. 39,
Issue. ,
p.
217.
Xiao, Yingqing
and
Yang, Fei
2017.
Singular Perturbations with Multiple Poles of the Simple Polynomials.
Qualitative Theory of Dynamical Systems,
Vol. 16,
Issue. 3,
p.
731.
XIAO, YINGQING
and
YANG, FEI
2017.
Singular perturbations of the unicritical polynomials with two parameters.
Ergodic Theory and Dynamical Systems,
Vol. 37,
Issue. 6,
p.
1997.
Boyd, Suzanne
and
Mitchell, Alexander J.
2023.
The Boundedness Locus and Baby Mandelbrot Sets for Some Generalized McMullen Maps.
International Journal of Bifurcation and Chaos,
Vol. 33,
Issue. 09,
Boyd, Suzanne
and
Hoeppner, Matthew
2025.
Baby Mandelbrot Sets and Spines in Some One-Dimensional Subspaces of the Parameter Space for Generalized McMullen Maps.
Qualitative Theory of Dynamical Systems,
Vol. 24,
Issue. 4,