No CrossRef data available.
Article contents
On the entropy of actions of nilpotent Lie groups and their lattice subgroups
Published online by Cambridge University Press: 21 October 2011
Abstract
We consider a natural class 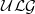 of connected, simply connected nilpotent Lie groups which contains ℝn, the group
of connected, simply connected nilpotent Lie groups which contains ℝn, the group 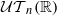 of all triangular unipotent matrices over ℝ and many of its subgroups, and is closed under direct products. If
of all triangular unipotent matrices over ℝ and many of its subgroups, and is closed under direct products. If 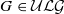 , then
, then 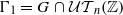 is a lattice subgroup of G. We prove that if
is a lattice subgroup of G. We prove that if 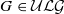 and Γ is a lattice subgroup of G, then a free ergodic measure-preserving action T of G on a probability space (X,ℬ,μ)has completely positive entropy (CPE) if and only if the restriction TΓ of T to Γ has CPE. We can deduce from this the following version of a well-known conjecture in this case: the action T has CPE if and only if T is uniformly mixing. Moreover, such T has a Lebesgue spectrum with infinite multiplicity. We further consider an ergodic free action T with positive entropy and suppose TΓ is ergodic for any lattice subgroup Γ of G. This holds, in particular, if the spectrum of T does not contain a discrete component. Then we show the Pinsker algebra Π(T)of T exists and coincides with the Pinsker algebras Π(TΓ)of TΓ for any lattice subgroup Γ of G. In this case, T always has Lebesgue spectrum with infinite multiplicity on the space ℒ20(X,μ)⊖ℒ20(Π(T)) , where ℒ20(Π(T))contains all Π(T) -measurable functions from ℒ20(X,μ) . To prove these results, we use the following formula: h(T)=∣G(Γ)∣−1hK (TΓ) , where h(T)is the Ornstein–Weiss entropy of T, hK (TΓ)is a Kolmogorov–Sinai entropy of TΓ, and the number ∣G(TΓ)∣ is the Haar measure of the compact subset G(Γ)of G. In particular, h(T)=hK (TΓ1) , and hK (TΓ1)=∣G(Γ)∣−1hK (TΓ) . The last relation is an analogue of the Abramov formula for flows.
and Γ is a lattice subgroup of G, then a free ergodic measure-preserving action T of G on a probability space (X,ℬ,μ)has completely positive entropy (CPE) if and only if the restriction TΓ of T to Γ has CPE. We can deduce from this the following version of a well-known conjecture in this case: the action T has CPE if and only if T is uniformly mixing. Moreover, such T has a Lebesgue spectrum with infinite multiplicity. We further consider an ergodic free action T with positive entropy and suppose TΓ is ergodic for any lattice subgroup Γ of G. This holds, in particular, if the spectrum of T does not contain a discrete component. Then we show the Pinsker algebra Π(T)of T exists and coincides with the Pinsker algebras Π(TΓ)of TΓ for any lattice subgroup Γ of G. In this case, T always has Lebesgue spectrum with infinite multiplicity on the space ℒ20(X,μ)⊖ℒ20(Π(T)) , where ℒ20(Π(T))contains all Π(T) -measurable functions from ℒ20(X,μ) . To prove these results, we use the following formula: h(T)=∣G(Γ)∣−1hK (TΓ) , where h(T)is the Ornstein–Weiss entropy of T, hK (TΓ)is a Kolmogorov–Sinai entropy of TΓ, and the number ∣G(TΓ)∣ is the Haar measure of the compact subset G(Γ)of G. In particular, h(T)=hK (TΓ1) , and hK (TΓ1)=∣G(Γ)∣−1hK (TΓ) . The last relation is an analogue of the Abramov formula for flows.
Information
- Type
- Research Article
- Information
- Ergodic Theory and Dynamical Systems , Volume 32 , Issue 2: Daniel J. Rudolph – in Memoriam , April 2012 , pp. 535 - 573
- Copyright
- Copyright © Cambridge University Press 2011

