Hostname: page-component-8448b6f56d-c47g7 Total loading time: 0 Render date: 2024-04-23T20:47:25.169Z Has data issue: false hasContentIssue false
  • English
  • Français

A REFINED WARING PROBLEM FOR FINITE SIMPLE GROUPS

Part of: Representation theory of groups

Published online by Cambridge University Press:  17 March 2015

MICHAEL LARSEN  and
PHAM HUU TIEP
MICHAEL LARSEN
Affiliation:
Department of Mathematics, Indiana University, Bloomington, IN 47405, USA; mjlarsen@indiana.edu
PHAM HUU TIEP
Affiliation:
Department of Mathematics, University of Arizona, Tucson, AZ 85721, USA; tiep@math.arizona.edu

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 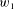$w_{1}$ and 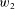$w_{2}$ be nontrivial words in free groups 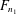$F_{n_{1}}$ and 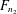$F_{n_{2}}$, respectively. We prove that, for all sufficiently large finite nonabelian simple groups $G$, there exist subsets 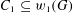$C_{1}\subseteq w_{1}(G)$ and 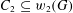$C_{2}\subseteq w_{2}(G)$ such that 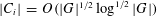$|C_{i}|=O(|G|^{1/2}\log ^{1/2}|G|)$ and $C_{1}C_{2}=G$. In particular, if $w$ is any nontrivial word and 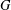$G$ is a sufficiently large finite nonabelian simple group, then 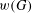$w(G)$ contains a thin base of order 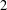$2$. This is a nonabelian analog of a result of Van Vu [‘On a refinement of Waring’s problem’, Duke Math. J. 105(1) (2000), 107–134.] for the classical Waring problem. Further results concerning thin bases of 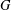$G$ of order 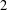$2$ are established for any finite group and for any compact Lie group 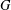$G$.

MSC classification

Primary: 20C33: Representations of finite groups of Lie type
Secondary: 20D06: Simple groups
Type
Research Article
Information
Forum of Mathematics, Sigma , Volume 3 , 2015 , e6
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/3.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author(s) 2015

References

Bourbaki, N., ‘Éléments de Mathématique. Fasc. XXXVII’, in: Groupes et Algèbres de Lie. Chapitre II: Algèbres de Lie Libres. Chapitre III: Groupes de Lie, Actualités Scientifiques et Industrielles, No. 1349 (Hermann, Paris, 1972).Google Scholar
Burkhardt, R., ‘Über die Zerlegungszahlen der Suzukigruppen Sz(q)’, J. Algebra 59 (1979), 421–433.Google Scholar
Conway, J. H., Curtis, R. T., Norton, S. P., Parker, R. A. and Wilson, R. A., An ATLAS of Finite Groups (Clarendon, Oxford, 1985).Google Scholar
Deligne, P. and Lusztig, G., ‘Representations of reductive groups over finite fields’, Ann. of Math. (2) 103(1) (1976), 103–161.CrossRefGoogle Scholar
Ellers, E. W. and Gordeev, N., ‘On the conjectures of J. Thompson and O. Ore’, Trans. Amer. Math. Soc. 350 (1998), 3657–3671.CrossRefGoogle Scholar
Fulman, J. and Guralnick, R. M., ‘Bounds on the number and sizes of conjugacy classes in finite Chevalley groups with applications to derangements’, Trans. Amer. Math. Soc. 364 (2012), 3023–3070.Google Scholar
Garion, S., Larsen, M. and Lubotzky, A., ‘Beauville surfaces and finite simple groups’, J. Reine Angew. Math. 666 (2012), 225–243.Google Scholar
Geck, M., Hiss, G., Lübeck, F., Malle, G. and Pfeiffer, G., ‘CHEVIE—A system for computing and processing generic character tables for finite groups of Lie type, Weyl groups and Hecke algebras’, Appl. Algebra Engrg. Comm. Comput. 7 (1996), 175–210.Google Scholar
Guralnick, R. M. and Lübeck, F., ‘On p-singular elements in Chevalley groups in characteristic p’, in: Groups and Computation, III, Vol. 8 (Columbus, OH, 1999) (Ohio State University Mathematical Research Institute Publication, de Gruyter, Berlin, 2001), 169–182.Google Scholar
Guralnick, R. M. and Tiep, P. H., ‘Effective results on the Waring problem for finite simple groups’, submitted.Google Scholar
Kleidman, P. B. and Liebeck, M. W., The Subgroup Structure of the Finite Classical Groups, London Mathematical Society Lecture Note Series. no. 129 (Cambridge University Press, 1990).Google Scholar
Larsen, M., ‘Word maps have large image’, Israel J. Math. 139 (2004), 149–156.Google Scholar
Larsen, M. and Shalev, A., ‘Characters of symmetric groups: sharp bounds and applications’, Invent. Math. 174(3) (2008), 645–687.Google Scholar
Larsen, M. and Shalev, A., ‘Word maps and Waring type problems’, J. Amer. Math. Soc. 22(2) (2009), 437–466.Google Scholar
Larsen, M., Shalev, A. and Tiep, P. H., ‘Waring problem for finite simple groups’, Ann. of Math. (2) 174(3) (2011), 1885–1950.Google Scholar
Liebeck, M. W., O’Brien, E., Shalev, A. and Tiep, P. H., ‘The ore conjecture’, J. Eur. Math. Soc. 12 (2010), 939–1008.Google Scholar
Liebeck, M. W. and Shalev, A., ‘Fuchsian groups, coverings of Riemann surfaces, subgroup growth, random quotients and random walks’, J. Algebra 276 (2004), 552–601.Google Scholar
Lübeck, F., ‘Finding p ′ -elements in finite groups of Lie type’, in: Groups and Computation, III, Vol. 8 (Columbus, OH, 1999) (Ohio State University Mathematical Research Institute Publication, de Gruyter, Berlin, 2001), 249–255.Google Scholar
Lübeck, F., Character degrees and their multiplicities for some groups of Lie type of rank ${<}9$, http://www.math.rwth-aachen.de/∼Frank.Luebeck/chev/DegMult/index.html.Google Scholar
Lübeck, F. and Malle, G., ‘(2, 3)-generation of exceptional groups’, J. Lond. Math. Soc. 59 (1999), 109–122.Google Scholar
Magaard, K., Malle, G. and Tiep, P. H., ‘Irreducibility of tensor squares, symmetric squares, and alternating squares’, Pacific J. Math. 202 (2002), 379–427.Google Scholar
Malle, G., Saxl, J. and Weigel, T., ‘Generation of classical groups’, Geom. Dedicata 49 (1994), 85–116.Google Scholar
Mattila, P., Geometry of Sets and Measures in Euclidean Spaces. Fractals and Rectifiability, Cambridge Studies in Advanced Mathematics, 44 (Cambridge University Press, Cambridge, 1995).Google Scholar
Tao, T., 245C, Notes 5: Hausdorff dimension, http://terrytao.wordpress.com/2009/05/19/245c-notes-5-hausdorff-dimension-optional/.Google Scholar
Van Vu, H., ‘On a refinement of Waring’s problem’, Duke Math. J. 105(1) (2000), 107–134.Google Scholar
Ward, H. N., ‘On Ree’s series of simple groups’, Trans. Amer. Math. Soc. 121 (1966), 62–89.Google Scholar