Article contents
The symplectic geometry of higher Auslander algebras: Symmetric products of disks
Published online by Cambridge University Press: 01 February 2021
Abstract
We show that the perfect derived categories of Iyama’s d-dimensional Auslander algebras of type 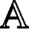 ${\mathbb {A}}$ are equivalent to the partially wrapped Fukaya categories of the d-fold symmetric product of the
${\mathbb {A}}$ are equivalent to the partially wrapped Fukaya categories of the d-fold symmetric product of the 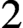 $2$-dimensional unit disk with finitely many stops on its boundary. Furthermore, we observe that Koszul duality provides an equivalence between the partially wrapped Fukaya categories associated to the d-fold symmetric product of the disk and those of its
$2$-dimensional unit disk with finitely many stops on its boundary. Furthermore, we observe that Koszul duality provides an equivalence between the partially wrapped Fukaya categories associated to the d-fold symmetric product of the disk and those of its 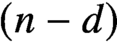 $(n-d)$-fold symmetric product; this observation leads to a symplectic proof of a theorem of Beckert concerning the derived Morita equivalence between the corresponding higher Auslander algebras of type
$(n-d)$-fold symmetric product; this observation leads to a symplectic proof of a theorem of Beckert concerning the derived Morita equivalence between the corresponding higher Auslander algebras of type 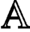 ${\mathbb {A}}$. As a by-product of our results, we deduce that the partially wrapped Fukaya categories associated to the d-fold symmetric product of the disk organise into a paracyclic object equivalent to the d-dimensional Waldhausen
${\mathbb {A}}$. As a by-product of our results, we deduce that the partially wrapped Fukaya categories associated to the d-fold symmetric product of the disk organise into a paracyclic object equivalent to the d-dimensional Waldhausen  $\text {S}_{\bullet }$-construction, a simplicial space whose geometric realisation provides the d-fold delooping of the connective algebraic K-theory space of the ring of coefficients.
$\text {S}_{\bullet }$-construction, a simplicial space whose geometric realisation provides the d-fold delooping of the connective algebraic K-theory space of the ring of coefficients.
Information
- Type
- Algebra
- Information
- Creative Commons
- This is an Open Access article, distributed under the terms of the Creative Commons Attribution-NonCommercial-ShareAlike licence (http://creativecommons.org/licenses/by-nc-sa/4.0/), which permits non-commercial re-use, distribution, and reproduction in any medium, provided the same Creative Commons licence is included and the original work is properly cited. The written permission of Cambridge University Press must be obtained for commercial re-use.
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
- 10
- Cited by


