Hostname: page-component-76fb5796d-zzh7m Total loading time: 0 Render date: 2024-04-27T01:04:33.541Z Has data issue: false hasContentIssue false
  • English
  • Français

Analysis of a non-reversible Markov chain speedup by a single edge

Part of: Markov processes Ergodic theory

Published online by Cambridge University Press:  04 January 2023

Balázs Gerencsér Open the ORCID record for Balázs Gerencsér [Opens in a new window]
Balázs Gerencsér*
Affiliation:
Alfréd Rényi Institute of Mathematics and ELTE Eötvös Loránd University
*
*Postal address: Alfréd Rényi Institute of Mathematics, 1053 Budapest, Reáltanoda utca 13-15, Hungary. Email address: gerencser.balazs@renyi.hu
Get access Rights & Permissions [Opens in a new window]

Abstract

We present a Markov chain example where non-reversibility and an added edge jointly improve mixing time. When a random edge is added to a cycle of n vertices and a Markov chain with a drift is introduced, we get a mixing time of 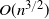$O(n^{3/2})$ with probability bounded away from 0. If only one of the two modifications were performed, the mixing time would stay $\Omega(n^2)$ .

Keywords

Mixing timerandom edgedrift

MSC classification

Primary: 60J10: Markov chains (discrete-time Markov processes on discrete state spaces)
Secondary: 37A25: Ergodicity, mixing, rates of mixing
Type
Original Article
Information
Journal of Applied Probability , Volume 60 , Issue 2 , June 2023 , pp. 642 - 660
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of Applied Probability Trust

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aldous, D. and Fill, J. A. (2002). Reversible Markov Chains and Random Walks on Graphs. Unfinished monograph, recompiled 2014, available at http://www.stat.berkeley.edu/~aldous/RWG/book.html.Google Scholar
Angel, O., Peres, Y. and Wilson, D. B. (2008). Card shuffling and Diophantine approximation. Ann. Appl. Prob. 18, 1215–1231.CrossRefGoogle Scholar
Berry, A. C. (1941). The accuracy of the Gaussian approximation to the sum of independent variates. Trans. Amer. Math. Soc. 49, 122–136.CrossRefGoogle Scholar
Boczkowski, L., Peres, Y. and Sousi, P. (2018). Sensitivity of mixing times in eulerian digraphs. SIAM J. Discrete Math. 32, 624–655.CrossRefGoogle Scholar
Boyd, S., Diaconis, P., Parrilo, P. and Xiao, L. (2009). Fastest mixing Markov chain on graphs with symmetries. SIAM J. Optim. 20, 792–819.CrossRefGoogle Scholar
Diaconis, P. (2009). The Markov chain Monte Carlo revolution. Bull. Amer. Math. Soc. 46, 179–205.CrossRefGoogle Scholar
Diaconis, P., Holmes, S. and Neal, R. M. (2000). Analysis of a nonreversible Markov chain sampler. Ann. Appl. Prob. 10, 726–752.CrossRefGoogle Scholar
Esseen, C.-G. (1942). On the Liapunov limit error in the theory of probability. Arkiv für Matematik, Astronomi och Fysik 28, 1–19.Google Scholar
Gerencsér, B. (2011). Markov chain mixing time on cycles. Stoch. Process. Appl. 121, 2553–2570.CrossRefGoogle Scholar
Gerencsér, B. and Hendrickx, J. M. (2019). Improved mixing rates of directed cycles by added connection. J. Theoret. Prob. 32, 684–701.CrossRefGoogle Scholar
Jarník, V. (1928–29). Zur metrischen Theorie der diophantischen Approximationen. Prace Matematyczno-Fizyczne 36, 91–106.Google Scholar
Khintchine, A. (1924). Einige Sätze über Kettenbrüche, mit Anwendungen auf die Theorie der diophantischen Approximationen. Mathematische Annalen 92, 115–125.CrossRefGoogle Scholar
Levin, D. A. and Peres, Y. (2017). Markov Chains and Mixing Times, 2nd edn. American Mathematical Society, Providence, RI.CrossRefGoogle Scholar
Spencer, J. (2014). Asymptopia. American Mathematical Society, Providence, RI.CrossRefGoogle Scholar
Walfisz, A. (1963). Weylsche exponentialsummen in der neueren zahlentheorie. VEB Deutscher Verlag der Wissenschaften, Berlin.Google Scholar