Hostname: page-component-8448b6f56d-sxzjt Total loading time: 0 Render date: 2024-04-23T13:37:14.202Z Has data issue: false hasContentIssue false
  • English
  • Français

Generation of acoustic tones in round jets at a Mach number of 0.9 impinging on a plate with and without a hole

Published online by Cambridge University Press:  11 February 2022

Mathieu Varé Open the ORCID record for Mathieu Varé [Opens in a new window]  and
Christophe Bogey Open the ORCID record for Christophe Bogey [Opens in a new window]
Mathieu Varé*
Affiliation:
Univ Lyon, École Centrale de Lyon, CNRS, Univ Claude Bernard Lyon 1, INSA Lyon, Laboratoire de Mécanique des Fluides et d'Acoustique, UMR5509, 69130 Ecully, France
Christophe Bogey
Affiliation:
Univ Lyon, École Centrale de Lyon, CNRS, Univ Claude Bernard Lyon 1, INSA Lyon, Laboratoire de Mécanique des Fluides et d'Acoustique, UMR5509, 69130 Ecully, France
*
†Email address for correspondence: mathieu.vare@ec-lyon.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

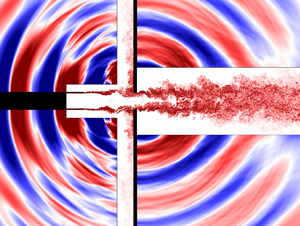
The generation of acoustic tones in four round jets at a Mach number of 0.9 impinging on a plate at a distance $L=6r_0$ from the nozzle exit, where $r_0$ is the nozzle radius, has been investigated by large-eddy simulation. Three plates are perforated by holes of diameters $h=2r_0$, $3r_0$ and $4.4r_0$, centred on the jet axis, whereas the fourth plate has no hole, in order to study the effects of the hole on the jet flow and acoustic fields. In all cases, acoustic tones emerge in the jet near field, upstream but also downstream of the plate for the perforated plates. Their frequencies are similar for all jets and close to those predicted for aeroacoustic feedback loops establishing between the nozzle and the plate, involving flow disturbances convected downstream and waves propagating upstream at the ambient speed of sound. Their levels, however, decrease with the hole diameter, by a few dB for $h\leqslant 3r_0$ but approximately by 40 dB for $h=4.4r_0$. The features of the feedback loops are identified by computing two-dimensional space–time correlations and frequency–wavenumber spectra of the pressure fluctuations in the jet mixing layers. These loops are found to be closed by free-stream upstream-propagating guided jet waves, produced by the impingement of vortical structures on the plate for $h\leqslant 3r_0$ and by the scattering of the jet aerodynamic pressure fluctuations by the hole edges for $h=4.4r_0$. Finally, an acoustic analogy is used to show that the contributions of the pressure fluctuations on the plate to the radiated noise are dominant.

JFM classification

Acoustics: Aeroacoustics Acoustics: Jet noise
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 936 , 10 April 2022 , A16
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Berland, J., Bogey, C., Marsden, O. & Bailly, C. 2007 High-order, low dispersive and low dissipative explicit schemes for multiple-scale and boundary problems. J. Comput. Phys. 224 (2), 637–662.CrossRefGoogle Scholar
Bogey, C. 2018 Grid sensitivity of flow field and noise of high-Reynolds-number jets computed by large-eddy simulation. Intl J. Aeroacoust. 17 (4–5), 399–424.CrossRefGoogle Scholar
Bogey, C. 2019 Two-dimensional features of correlations in the flow and near pressure fields of Mach number 0.9 jets. AIAA Paper 2019-0806.CrossRefGoogle Scholar
Bogey, C. 2021 a Acoustic tones in the near-nozzle region of jets: characteristics and variations between Mach numbers 0.5 and 2. J. Fluid Mech. 921, A3.CrossRefGoogle Scholar
Bogey, C. 2021 b Tones in the acoustic far field of jets in the upstream direction. AIAA J. https://doi.org/10.2514/1.J061013.CrossRefGoogle Scholar
Bogey, C. & Bailly, C. 2002 Three-dimensional non-reflective boundary conditions for acoustic simulations: far-field formulation and validation test cases. Acta Acust. United Acust. 88 (4), 463–471.Google Scholar
Bogey, C. & Bailly, C. 2004 A family of low dispersive and low dissipative explicit schemes for flow and noise computations. J. Comput. Phys. 194 (1), 194–214.CrossRefGoogle Scholar
Bogey, C. & Bailly, C. 2006 Large-eddy simulations of transitional round jets: influence of the Reynolds number on flow development and energy dissipation. Phys. Fluids 18 (6), 065101.CrossRefGoogle Scholar
Bogey, C., De Cacqueray, N. & Bailly, C. 2011 a Finite differences for coarse azimuthal discretization and for reduction of effective resolution near origin of cylindrical flow equations. J. Comput. Phys. 230 (4), 1134–1146.CrossRefGoogle Scholar
Bogey, C. & Gojon, R. 2017 Feedback loop and upwind-propagating waves in ideally expanded supersonic impinging round jets. J. Fluid Mech. 823, 562–591.CrossRefGoogle Scholar
Bogey, C., Marsden, O. & Bailly, C. 2011 b Large-eddy simulation of the flow and acoustic fields of a Reynolds number $10^{5}$ subsonic jet with tripped exit boundary layers. Phys. Fluids 23 (3), 035104.CrossRefGoogle Scholar
Brès, G., Jordan, P., Jaunet, V., Le Rallic, M., Cavalieri, A., Towne, A., Lele, S., Colonius, T. & Schmidt, O. 2018 Importance of the nozzle-exit boundary-layer state in subsonic turbulent jets. J. Fluid Mech. 851, 83–124.CrossRefGoogle Scholar
Buchmann, N.A., Mitchell, D.M., Ingvorsen, K.M., Honnery, D.R. & Soria, J. 2011 High spatial resolution imaging of a supersonic underexpanded jet impinging on a flat plate. In Proceedings of the 6th Australian Conference on Laser Diagnostics in Fluid Mechanics and Combustion, vol. 116.Google Scholar
Chanaud, R.C. & Powell, A. 1965 Some experiments concerning the hole and ring tone. J. Acoust. Soc. Am. 37 (5), 902–911.CrossRefGoogle Scholar
Curle, N. 1955 The influence of solid boundaries upon aerodynamic sound. Proc. R. Soc. Lond. A 231 (1187), 505–514.Google Scholar
Dauptain, A., Gicquel, L.Y. & Moreau, S. 2012 Large-eddy simulation of supersonic impinging jets. AIAA J. 50 (7), 1560–1574.CrossRefGoogle Scholar
Edgington-Mitchell, D. 2019 Aeroacoustic resonance and self-excitation in screeching and impinging supersonic jets – a review. Intl J. Aeroacoust. 18 (2–3), 118–188.CrossRefGoogle Scholar
Edgington-Mitchell, D., Jaunet, V., Jordan, P., Towne, A., Soria, J. & Honnery, D. 2018 Upstream-travelling acoustic jet modes as a closure mechanism for screech. J. Fluid Mech. 855, R1.CrossRefGoogle Scholar
Fauconnier, D., Bogey, C. & Dick, E. 2013 On the performance of relaxation filtering for large-eddy simulation. J. Turbul. 14 (1), 22–49.CrossRefGoogle Scholar
Gloerfelt, X., Bailly, C. & Juvé, D. 2003 Direct computation of the noise radiated by a subsonic cavity flow and application of integral methods. J. Sound Vib. 266 (1), 119–146.CrossRefGoogle Scholar
Gloerfelt, X., Pérot, F., Bailly, C. & Juvé, D. 2005 Flow-induced cylinder noise formulated as a diffraction problem for low Mach numbers. J. Sound Vib. 287 (1–2), 129–151.CrossRefGoogle Scholar
Gojon, R. & Bogey, C. 2017 a Flow structure oscillations and tone production in underexpanded impinging round jets. AIAA J. 55 (6), 1792–1805.CrossRefGoogle Scholar
Gojon, R. & Bogey, C. 2017 b Numerical study of the flow and the near acoustic fields of an underexpanded round free jet generating two screech tones. Intl J. Aeroacoust. 16 (7–8), 603–625.CrossRefGoogle Scholar
Gojon, R. & Bogey, C. 2018 Flow features near plate impinged by ideally expanded and underexpanded round jets. AIAA J. 56 (2), 445–457.CrossRefGoogle Scholar
Gojon, R., Bogey, C. & Marsden, O. 2016 Investigation of tone generation in ideally expanded supersonic planar impinging jets using large-eddy simulation. J. Fluid Mech. 808, 90–115.CrossRefGoogle Scholar
Henderson, B., Bridges, J. & Wernet, M. 2005 An experimental study of the oscillatory flow structure of tone-producing supersonic impinging jets. J. Fluid Mech. 542, 115–137.CrossRefGoogle Scholar
Ho, C.-M. & Nosseir, N.S. 1981 Dynamics of an impinging jet. Part 1. The feedback phenomenon. J. Fluid Mech. 105, 119–142.CrossRefGoogle Scholar
Howe, M.S. 1979 On the theory of unsteady high Reynolds number flow through a circular aperture. Proc. R. Soc. Lond. A 366 (1725), 205–223.Google Scholar
Jaunet, V., Mancinelli, M., Jordan, P., Towne, A., Edgington-Mitchell, D.M., Lehnasch, G. & Girard, S. 2019 Dynamics of round jet impingement. AIAA Paper 2019-2769.CrossRefGoogle Scholar
Jordan, P., Jaunet, V., Towne, A., Cavalieri, A., Colonius, T., Schmidt, O. & Agarwal, A. 2018 Jet-flap interaction tones. J. Fluid Mech. 853, 333–358.CrossRefGoogle Scholar
Kawai, S., Tsutsumi, S., Takaki, R. & Fujii, K. 2007 Computational aeroacoustic analysis of overexpanded supersonic jet impingement on a flat plate with/without hole. In ASME/JSME 2007 5th Joint Fluids Engineering Conference 2007-37563, pp. 1163–1167.Google Scholar
Kremer, F. & Bogey, C. 2015 Large-eddy simulation of turbulent channel flow using relaxation filtering: resolution requirement and Reynolds number effects. Comput. Fluids 116, 17–28.CrossRefGoogle Scholar
Langthjem, M.A. & Nakano, M. 2005 The jet hole-tone oscillation cycle subjected to acoustic excitation: a numerical study based on an axisymmetric vortex method. In The Proceedings of the International Conference on Jets, Wakes and Separated Flows, vol. 2005, pp. 745–750.Google Scholar
Launder, B.E. & Rodi, W. 1983 The turbulent wall jet measurements and modeling. Annu. Rev. Fluid Mech. 15 (1), 429–459.CrossRefGoogle Scholar
Lawrence, J., Azarpeyvand, M. & Self, R. 2011 Interaction between a flat plate and a circular subsonic jet. AIAA Paper 2011-2745.CrossRefGoogle Scholar
Mancinelli, M., Jaunet, V., Jordan, P. & Towne, A. 2019 Screech-tone prediction using upstream-travelling jet modes. Exp. Fluids 60 (1), 22.CrossRefGoogle Scholar
Matsuura, K. & Nakano, M. 2012 A throttling mechanism sustaining a hole tone feedback system at very low Mach numbers. J. Fluid Mech. 710, 569–605.CrossRefGoogle Scholar
Meganathan, A. & Vakili, A. 2006 An experimental study of acoustic and flow characteristics of hole tones. AIAA Paper 2006-1015.CrossRefGoogle Scholar
Michalke, A. 1984 Survey on jet instability theory. Prog. Aerosp. Sci. 21, 159–199.CrossRefGoogle Scholar
Mitchell, D.M., Honnery, D.R. & Soria, J. 2012 The visualization of the acoustic feedback loop in impinging underexpanded supersonic jet flows using ultra-high frame rate schlieren. J. Vis. (Visualization) 15 (4), 333–341.CrossRefGoogle Scholar
Mohseni, K. & Colonius, T. 2000 Numerical treatment of polar coordinate singularities. J. Comput. Phys. 157 (2), 787–795.CrossRefGoogle Scholar
Neuwerth, G. 1974 Acoustic feedback of a subsonic and supersonic free jet which impinges on an obstacle. NASA Technical Translation F-15719.Google Scholar
Nogueira, P.A.S., Cavalieri, A.V.G & Jordan, P. 2017 A model problem for sound radiation by an installed jet. J. Sound Vib. 391, 95–115.CrossRefGoogle Scholar
Norum, T.D. 1991 Supersonic rectangular jet impingement noise experiments. AIAA J. 29 (7), 1051–1057.CrossRefGoogle Scholar
Nosseir, N.S. & Ho, C.-M. 1982 Dynamics of an impinging jet. Part 2. The noise generation. J. Fluid Mech. 116, 379–391.CrossRefGoogle Scholar
Panickar, P. & Raman, G. 2007 Criteria for the existence of helical instabilities in subsonic impinging jets. Phys. Fluids 19 (10), 103–106.CrossRefGoogle Scholar
Poreh, M., Tsuei, Y.G. & Cermak, J.E. 1967 Investigation of a turbulent radial wall jet. Trans. ASME J. Appl. Mech. 34 (2), 457–463.CrossRefGoogle Scholar
Powell, A. 1953 On edge tones and associated phenomena. Acta Acust. United Acust. 3 (4), 233–243.Google Scholar
Preisser, J.S. 1979 Fluctuating surface pressure and acoustic radiation for subsonic normal jet impingement. NASA Tech. Paper 1361.Google Scholar
Rayleigh, Lord 1945 The Theory of Sound, vols. I and II. Cambridge University Press.Google Scholar
Ricou, F.P. & Spalding, D.B. 1961 Measurements of entrainment by axisymmetrical turbulent jets. J. Fluid Mech. 11 (1), 21–32.CrossRefGoogle Scholar
Risborg, A. & Soria, J. 2009 High-speed optical measurements of an underexpanded supersonic jet impinging on an inclined plate. In Proceedings of SPIE 7126, 28th International Congress on High-Speed Imaging and Photonics. International Society for Optics and Photonics.CrossRefGoogle Scholar
Schmidt, O.T., Towne, A., Colonius, T., Cavalieri, A.V.G., Jordan, P. & Brès, G.A. 2017 Wavepackets and trapped acoustic modes in a turbulent jet: coherent structure eduction and global stability. J. Fluid Mech. 825, 1153–1181.CrossRefGoogle Scholar
Sondhauss, C. 1854 Über die beim Ausströmen der Luft entstehenden Töne. Ann. Phys. 167 (2), 214–240.CrossRefGoogle Scholar
Tam, C.K.W. & Ahuja, K.K. 1990 Theoretical model of discrete tone generation by impinging jets. J. Fluid Mech. 214, 67–87.CrossRefGoogle Scholar
Tam, C.K.W. & Chandramouli, S. 2020 Jet-plate interaction tones relevant to over-the-wing engine mount concept. J. Sound Vib. 486, 115378.CrossRefGoogle Scholar
Tam, C.K.W. & Dong, Z. 1996 Radiation and outflow boundary conditions for direct computation of acoustic and flow disturbances in a non uniform mean flow. J. Comput. Acoust. 4 (02), 175–201.CrossRefGoogle Scholar
Tam, C.K.W. & Norum, T.D. 1992 Impingement tones of large aspect ratio supersonic rectangular jets. AIAA J. 30 (2), 304–311.CrossRefGoogle Scholar
Towne, A., Cavalieri, A.V.G., Jordan, P., Colonius, T., Schmidt, O., Jaunet, V. & Brès, G.A. 2017 Acoustic resonance in the potential core of subsonic jets. J. Fluid Mech. 825, 1113–1152.CrossRefGoogle Scholar
Troyes, J.N., Vuillot, F., Langenais, A. & Lambaré, H. 2019 Coupled CFD-CAA simulation of the noise generated by a hot supersonic jet impinging on a flat plate with exhaust hole. AIAA Paper 2019-2752.CrossRefGoogle Scholar
Tsutsumi, S., Takaki, R., Ikaida, H. & Terashima, K. 2015 Numerical aeroacoustics analysis of a scaled solid jet impinging on flat plate with exhaust hole. In 30th International Symposium on Space Technology and Science.Google Scholar
Umeda, Y. & Ishii, R. 1993 Hole tone generation from highly choked jets. J. Acoust. Soc. Am. 94 (2), 1058–1066.CrossRefGoogle Scholar
Umeda, Y., Maeda, H. & Ishii, R. 1988 Hole tone generated from almost choked to highly choked jets. AIAA J. 26 (9), 1036–1043.CrossRefGoogle Scholar
Van Hout, R., Rinsky, V. & Grobman, Y.G. 2018 Experimental study of a round jet impinging on a flat surface: flow field and vortex characteristics in the wall jet. Intl J. Heat Fluid Flow 70, 41–58.CrossRefGoogle Scholar
Varé, M. & Bogey, C. 2021 Large-eddy simulations of the flow and acoustic fields of a rocket jet impinging on a perforated plate. AIAA Paper 2021-2152.CrossRefGoogle Scholar
Vinoth, B.R. & Rathakrishnan, E. 2011 Effect of impinging plate geometry on the self-excitation of subsonic impinging jets. J. Fluids Struct. 27 (8), 1238–1251.CrossRefGoogle Scholar

Varé and Bogey supplementary movie 1

Vorticity norm and pressure fluctuations for jetnohole.

Download Varé and Bogey supplementary movie 1(Video)
Video 6.4 MB

Varé and Bogey supplementary movie 2

Vorticity norm and pressure fluctuations for jetmediumhole.

Download Varé and Bogey supplementary movie 2(Video)
Video 10.9 MB

Varé and Bogey supplementary movie 3

Correlations of p'(r=1.5r0,z=0,t) with p'(r,z,t+δt) for nθ=1 for jetmediumhole. A circle centered on (z=L,r=1.5r0) is displayed as a black line.

Download Varé and Bogey supplementary movie 3(Video)
Video 1 MB

Varé and Bogey supplementary movie 4

Correlations of p'(r=1.5r0,z=0,t) with p'(r,z,t+δt) for nθ=1 for jetlargehole. A circle centered on (z=L,r=2.2r0) is displayed as a black line.

Download Varé and Bogey supplementary movie 4(Video)
Video 888 KB